Page 126 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 126
athan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
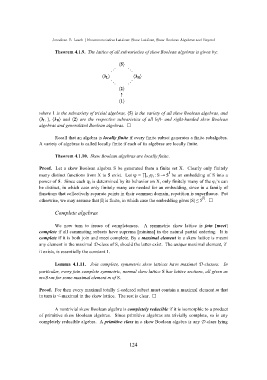
Theorem 4.1.9. The lattice of all subvarieties of skew Boolean algebras is given by:
〈5〉
〈3L〉 〈3R〉
〈2〉
↑
〈1〉
where 1 is the subvariety of trivial algebras, 〈5〉 is the variety of all skew Boolean algebras, and
〈3L 〉, 〈3R〉 and 〈2〉 are the respective subvarieties of all left- and right-handed skew Boolean
algebras and generalized Boolean algebras. £
Recall that an algebra is locally finite if every finite subset generates a finite subalgebra.
A variety of algebras is called locally finite if each of its algebras are locally finite.
Theorem 4.1.10. Skew Boolean algebras are locally finite.
Proof. Let a skew Boolean algebra S be generated from a finite set X. Clearly only finitely
many distinct functions from X to 5 exist. Let ϕ = ∏i∈Iϕi : S → 5I be an embedding of S into a
power of 5. Since each ϕi is determined by its behavior on X, only finitely many of the ϕi’s can
be distinct, in which case only finitely many are needed for an embedding, since in a family of
functions that collectively separate points in their common domain, repetition is superfluous. Put
otherwise, we may assume that |I| is finite, in which case the embedding gives |S| ≤ 5|I|. £
Complete algebras
We now turn to issues of completeness. A symmetric skew lattice is join [meet]
complete if all commuting subsets have suprema [minima] in the natural partial ordering. It is
complete if it is both join and meet complete. By a maximal element in a skew lattice is meant
any element in the maximal D-class of S, should the latter exist. The unique maximal element, if
it exists, is essentially the constant 1.
Lemma 4.1.11. Join complete, symmetric skew lattices have maximal D-classes. In
particular, every join complete symmetric, normal skew lattice S has lattice sections, all given as
m∧S∧m for some maximal element m of S.
Proof. For then every maximal totally ≤-ordered subset must contain a maximal element m that
in turn is ≤-maximal in the skew lattice. The rest is clear. £
A nontrivial skew Boolean algebra is completely reducible if it is isomorphic to a product
of primitive skew Boolean algebras. Since primitive algebras are trivially complete, so is any
completely reducible algebra. A primitive class in a skew Boolean algebra is any D-class lying
124
Theorem 4.1.9. The lattice of all subvarieties of skew Boolean algebras is given by:
〈5〉
〈3L〉 〈3R〉
〈2〉
↑
〈1〉
where 1 is the subvariety of trivial algebras, 〈5〉 is the variety of all skew Boolean algebras, and
〈3L 〉, 〈3R〉 and 〈2〉 are the respective subvarieties of all left- and right-handed skew Boolean
algebras and generalized Boolean algebras. £
Recall that an algebra is locally finite if every finite subset generates a finite subalgebra.
A variety of algebras is called locally finite if each of its algebras are locally finite.
Theorem 4.1.10. Skew Boolean algebras are locally finite.
Proof. Let a skew Boolean algebra S be generated from a finite set X. Clearly only finitely
many distinct functions from X to 5 exist. Let ϕ = ∏i∈Iϕi : S → 5I be an embedding of S into a
power of 5. Since each ϕi is determined by its behavior on X, only finitely many of the ϕi’s can
be distinct, in which case only finitely many are needed for an embedding, since in a family of
functions that collectively separate points in their common domain, repetition is superfluous. Put
otherwise, we may assume that |I| is finite, in which case the embedding gives |S| ≤ 5|I|. £
Complete algebras
We now turn to issues of completeness. A symmetric skew lattice is join [meet]
complete if all commuting subsets have suprema [minima] in the natural partial ordering. It is
complete if it is both join and meet complete. By a maximal element in a skew lattice is meant
any element in the maximal D-class of S, should the latter exist. The unique maximal element, if
it exists, is essentially the constant 1.
Lemma 4.1.11. Join complete, symmetric skew lattices have maximal D-classes. In
particular, every join complete symmetric, normal skew lattice S has lattice sections, all given as
m∧S∧m for some maximal element m of S.
Proof. For then every maximal totally ≤-ordered subset must contain a maximal element m that
in turn is ≤-maximal in the skew lattice. The rest is clear. £
A nontrivial skew Boolean algebra is completely reducible if it is isomorphic to a product
of primitive skew Boolean algebras. Since primitive algebras are trivially complete, so is any
completely reducible algebra. A primitive class in a skew Boolean algebra is any D-class lying
124