Page 132 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 132
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
universal homomorphism from A to W. Then if A is a free V-algebra on generating set X, then
A/θ is a free W-algebra on generating set ϕA[X]. In the above context, θA = D, L or R as
appropriate, with X and ϕA[X] being equipotent under ϕA.)
In what follows we first consider SBAn, LSBAn, etc. which denote SBAX, LSBAX etc. on
alphabet X = {x1, x2, … , xn}. Their standard atomic decomposition is given in Theorem 4.2.6
below. But to obtain the latter we need to understand their atomic structure. The case for LSBAn
and for RSBAn is described in Theorem 4.2.5, the content of which is our immediate goal. We
focus on LSBAn. Since LSBAn has finitely many generators it will be finite and thus is
determined by its atomic D-classes, all lying just above the class {0}. We first describe these
classes. Each class consists of atoms all sharing a common form. The justification that they are
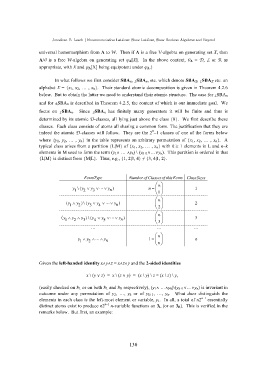
indeed the atomic D-classes will follow. They are the 2n–1 classes of one of the forms below
where {y1, y2, … , yn} in the table represents an arbitrary permutation of {x1, x2, … , xn}. A
typical class arises from a partition {L|M} of {x1, x2, … , xn} with k ≥ 1 elements in L and n–k
elements in M used to form the term (y1∧ … ∧yk) \ (yk+1∨…∨yn). This partition is ordered in that
{L|M} is distinct from {M|L}. Thus, e.g., {1, 2|3, 4} ≠ {3, 4|1, 2}.
FormType Number of Classes of this Form Class Sizes
y1 \ (y2 ∨ y3 ∨ ∨ yn ) n = ⎛ n⎞ 1
⎜⎝ 1 ⎠⎟
(y1 ∧ y2 ) \ (y3 ∨ y4 ∨ ∨ yn ) ⎛ n⎞ 2
⎜⎝ 2⎠⎟
(y1 ∧ y2 ∧ y3) \ (y4 ∨ y5 ∨ ∨ yn ) ⎛ n⎞ 3
⎜⎝ 3⎠⎟
y1 ∧ y2 ∧ ∧ yn 1 = ⎛ n⎞ n
⎜⎝ n⎠⎟
Given the left-handed identity x∧y∧z = x∧z∧y and the 2-sided identities
x \ (y ∨ z) = x \ (z ∨ y) = (x \ y) \ z = (x \ z) \ y,
(easily checked on 3L or on both 3L and 3R respectively), (y1∧ …∧yk)\(yk+1∨…∨yn) is invariant in
outcome under any permutation of y2, …, yk or of yk+1, …, yn. What does distinguish the
elements in each class is the left-most element or variable, y1. In all, a total of n2n–1 essentially
distinct atoms exist to produce n2n–1 n-variable functions on 3L (or on 3R). This is verified in the
remarks below. But first, an example:
130
universal homomorphism from A to W. Then if A is a free V-algebra on generating set X, then
A/θ is a free W-algebra on generating set ϕA[X]. In the above context, θA = D, L or R as
appropriate, with X and ϕA[X] being equipotent under ϕA.)
In what follows we first consider SBAn, LSBAn, etc. which denote SBAX, LSBAX etc. on
alphabet X = {x1, x2, … , xn}. Their standard atomic decomposition is given in Theorem 4.2.6
below. But to obtain the latter we need to understand their atomic structure. The case for LSBAn
and for RSBAn is described in Theorem 4.2.5, the content of which is our immediate goal. We
focus on LSBAn. Since LSBAn has finitely many generators it will be finite and thus is
determined by its atomic D-classes, all lying just above the class {0}. We first describe these
classes. Each class consists of atoms all sharing a common form. The justification that they are
indeed the atomic D-classes will follow. They are the 2n–1 classes of one of the forms below
where {y1, y2, … , yn} in the table represents an arbitrary permutation of {x1, x2, … , xn}. A
typical class arises from a partition {L|M} of {x1, x2, … , xn} with k ≥ 1 elements in L and n–k
elements in M used to form the term (y1∧ … ∧yk) \ (yk+1∨…∨yn). This partition is ordered in that
{L|M} is distinct from {M|L}. Thus, e.g., {1, 2|3, 4} ≠ {3, 4|1, 2}.
FormType Number of Classes of this Form Class Sizes
y1 \ (y2 ∨ y3 ∨ ∨ yn ) n = ⎛ n⎞ 1
⎜⎝ 1 ⎠⎟
(y1 ∧ y2 ) \ (y3 ∨ y4 ∨ ∨ yn ) ⎛ n⎞ 2
⎜⎝ 2⎠⎟
(y1 ∧ y2 ∧ y3) \ (y4 ∨ y5 ∨ ∨ yn ) ⎛ n⎞ 3
⎜⎝ 3⎠⎟
y1 ∧ y2 ∧ ∧ yn 1 = ⎛ n⎞ n
⎜⎝ n⎠⎟
Given the left-handed identity x∧y∧z = x∧z∧y and the 2-sided identities
x \ (y ∨ z) = x \ (z ∨ y) = (x \ y) \ z = (x \ z) \ y,
(easily checked on 3L or on both 3L and 3R respectively), (y1∧ …∧yk)\(yk+1∨…∨yn) is invariant in
outcome under any permutation of y2, …, yk or of yk+1, …, yn. What does distinguish the
elements in each class is the left-most element or variable, y1. In all, a total of n2n–1 essentially
distinct atoms exist to produce n2n–1 n-variable functions on 3L (or on 3R). This is verified in the
remarks below. But first, an example:
130