Page 16 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 16
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
showing that ≥ is transitive. L3 yields the basic duality x ∧ y = y if and only if x ∨ y = y. (L; ≥) is
indeed a poset. Its meets and joins are given by ∧ and ∨. As stated above, L0 is redundant
relative to L1-L3. Indeed:
Lemma 1.1.1. Given binary operations ∧ and ∨ on a set L, L3 implies L0.
Proof. Given L3, x ∧ x = x ∧ [x ∨ (x∧x)] = x, and thus x ∨ x = x ∨ (x ∧ x) = x. £
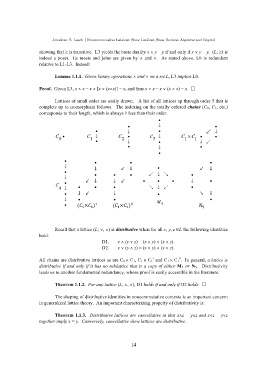
Lattices of small order are easily drawn. A list of all lattices up through order 5 that is
complete up to isomorphism follows. The indexing on the totally ordered chains (C0, C1, etc.)
corresponds to their length, which is always 1 less than their order.
•
•↓ •
C0 • • ↓• ↙↓
C1 C2 • C3 ↓ C1 × C1 •
↓ • •
• ↓ ↓
↙
•↓
•
•
• • • •
↓ ↙↓ ↙↓
↓ • •• • •
↙↓ ↓↙ ↓ ↓•
• •• • ••• •
↓↙ ↓ ↓ ↘↓
↓ • •
(!×!)! (!×!)! • •
C4 • !
↓ M5
•
↓
•
hold: Recall that a lattice (L; ∨, ∧) is distributive when for all x, y, z ∈L the following identities
D1. x ∧ (y ∨ z) = (x ∧ y) ∨ (x ∧ z).
D2. x ∨ (y ∧ z) = (x ∨ y) ∧ (x ∨ z).
All chains are distributive lattices as are C1 × C1, C1 × C1I and C1 × C10. In general, a lattice is
distributive if and only if it has no sublattice that is a copy of either M5 or N5. Distributivity
leads us to another fundamental redundancy, whose proof is easily accessible in the literature.
Theorem 1.1.2. For any lattice (L, ∧, ∨), D1 holds if and only if D2 holds. £
The shaping of distributive identities in noncommutative contexts is an important concern
in generalized lattice theory. An important characterizing property of distributivity is:
Theorem 1.1.3. Distributive lattices are cancellative in that x∧z = y∧z and x∨z = y∨z
together imply x = y. Conversely, cancellative skew lattices are distributive.
14
showing that ≥ is transitive. L3 yields the basic duality x ∧ y = y if and only if x ∨ y = y. (L; ≥) is
indeed a poset. Its meets and joins are given by ∧ and ∨. As stated above, L0 is redundant
relative to L1-L3. Indeed:
Lemma 1.1.1. Given binary operations ∧ and ∨ on a set L, L3 implies L0.
Proof. Given L3, x ∧ x = x ∧ [x ∨ (x∧x)] = x, and thus x ∨ x = x ∨ (x ∧ x) = x. £
Lattices of small order are easily drawn. A list of all lattices up through order 5 that is
complete up to isomorphism follows. The indexing on the totally ordered chains (C0, C1, etc.)
corresponds to their length, which is always 1 less than their order.
•
•↓ •
C0 • • ↓• ↙↓
C1 C2 • C3 ↓ C1 × C1 •
↓ • •
• ↓ ↓
↙
•↓
•
•
• • • •
↓ ↙↓ ↙↓
↓ • •• • •
↙↓ ↓↙ ↓ ↓•
• •• • ••• •
↓↙ ↓ ↓ ↘↓
↓ • •
(!×!)! (!×!)! • •
C4 • !
↓ M5
•
↓
•
hold: Recall that a lattice (L; ∨, ∧) is distributive when for all x, y, z ∈L the following identities
D1. x ∧ (y ∨ z) = (x ∧ y) ∨ (x ∧ z).
D2. x ∨ (y ∧ z) = (x ∨ y) ∧ (x ∨ z).
All chains are distributive lattices as are C1 × C1, C1 × C1I and C1 × C10. In general, a lattice is
distributive if and only if it has no sublattice that is a copy of either M5 or N5. Distributivity
leads us to another fundamental redundancy, whose proof is easily accessible in the literature.
Theorem 1.1.2. For any lattice (L, ∧, ∨), D1 holds if and only if D2 holds. £
The shaping of distributive identities in noncommutative contexts is an important concern
in generalized lattice theory. An important characterizing property of distributivity is:
Theorem 1.1.3. Distributive lattices are cancellative in that x∧z = y∧z and x∨z = y∨z
together imply x = y. Conversely, cancellative skew lattices are distributive.
14