Page 246 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 246
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
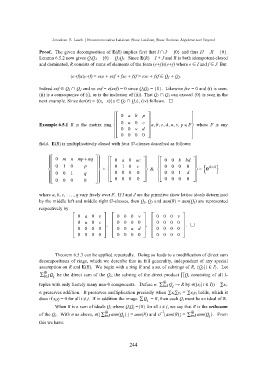
Proof. The given decomposition of E(R) implies first that I ∩ J = {0} and thus IJ = JI = {0}.
Lemma 6.5.2 now gives QIQJ = {0} = QJQI. Since E(R) = I + J and R is both idempotent-closed
and dominated, R consists of sums of elements of the form (e+f)x(e+f) where e ∈ I and f ∈ J. But
(e+f)x(e+f) = exe + exf + fxe + fxf = exe + fxf ∈ QI + QJ.
Indeed exf ∈ QI ∩ QJ and so exf = e(exf) = 0 since QIQJ = {0}. Likewise fxe = 0 and (i) is seen.
(ii) is a consequence of (i), as is the inclusion of (iii). That QI ∩ QJ can exceed {0} is seen in the
next example. Since ker(σ) = {(x, –x)⎪x ∈ QI ∩ QJ}, (iv) follows. £
Example 6.5.1 R is the matrix ring ⎧⎡ 0 a b p ⎤ ⎫ where F is any
⎪⎪⎨⎢⎢ ⎥ p ∈F ⎪⎪⎬
⎪⎢ 0 u 0 c ⎥ a, b, c, d, u, v,
0 0 v d ⎥ ⎪
⎪⎩⎣⎢ 0 0 0 0 ⎦⎥ ⎭⎪
field. E(R) is multiplicatively closed with four D-classes described as follows:
⎧⎡ 0 m n mp + nq ⎤⎫ ⎧⎡ 0 a 0 ac ⎤⎫ ⎧⎡ 0 0 b bd ⎤⎫
{ }⎪⎨⎪⎪⎪⎩⎢⎢⎢⎢⎣1 0 p ⎥⎥⎥⎦⎥⎪⎭⎪⎪⎪⎬ ⎪⎨⎪⎢⎢ 0 1 0 ⎥⎥⎪⎪⎬ & ⎪⎪⎨⎢⎢ 0 0 ⎥⎥⎬⎪⎪ >
0 0 1 q > ⎪⎢ 0 0 0 c ⎥⎪ ⎪⎢ 0 0 1 0 ⎥⎪ 04×4
0 0 0 0 ⎩⎪⎣⎢ 0 0 0 0 0 0 0 d
0
0 ⎥⎦⎭⎪ ⎪⎩⎢⎣ 0 0 ⎦⎥⎪⎭
where a, b, c, . . . , q vary freely over F. If I and J are the primitive skew lattice ideals determined
by the middle left and middle right D-classes, then QI, QJ and ann(R) = ann(QI) are represented
respectively by
⎡0 a 0 v⎤ ⎡0 0 b v⎤ ⎡0 0 0 v⎤
⎢ ⎥ ⎢ ⎥ ⎢ ⎥
⎢ 0 u 0 c ⎥, ⎢ 0 0 0 0 ⎥, ⎢ 0 0 0 0 ⎥. £
⎢ 0 0 0 0 ⎥ ⎢ 0 0 u d ⎥ ⎢ 0 0 0 0 ⎥
⎣⎢ 0 0 0 0 ⎦⎥ ⎢⎣ 0 0 0 0 ⎥⎦ ⎣⎢ 0 0 0 0 ⎦⎥
Theorem 6.5.3 can be applied repeatedly. Doing so leads to a modification of direct sum
decompositions of rings, which we describe this in full generality, independent of any special
assumption on R and E(R). We begin with a ring R and a set of subrings of R, {Qi⎪i ∈ I}. Let
∑i⊕∈I Qi be the direct sum of the Qi, the subring of the direct product ∏Qi consisting of all I-
tuples with only finitely many non-0 components. Define σ: ∑i⊕∈I Qi → R by σ(⟨xi⎪i ∈ I⟩) = ∑xi.
σ preserves addition. It preserves multiplication precisely when ∑xi∑yi = ∑xiyi holds, which it
does if xiyj = 0 for all i ≠ j. If in addition the image ∑ Qi = R, then each Qi must be an ideal of R.
When R is a sum of ideals Qi where QiQj ={0} for all i ≠ j, we say that R is the orthosum
of the Qi. With σ as above, σ([ ∑i⊕∈I ann(Qi ) ] = ann(R) and σ–1[ann(R)] = ∑i⊕∈I ann(Qi ) . From
this we have:
244
Proof. The given decomposition of E(R) implies first that I ∩ J = {0} and thus IJ = JI = {0}.
Lemma 6.5.2 now gives QIQJ = {0} = QJQI. Since E(R) = I + J and R is both idempotent-closed
and dominated, R consists of sums of elements of the form (e+f)x(e+f) where e ∈ I and f ∈ J. But
(e+f)x(e+f) = exe + exf + fxe + fxf = exe + fxf ∈ QI + QJ.
Indeed exf ∈ QI ∩ QJ and so exf = e(exf) = 0 since QIQJ = {0}. Likewise fxe = 0 and (i) is seen.
(ii) is a consequence of (i), as is the inclusion of (iii). That QI ∩ QJ can exceed {0} is seen in the
next example. Since ker(σ) = {(x, –x)⎪x ∈ QI ∩ QJ}, (iv) follows. £
Example 6.5.1 R is the matrix ring ⎧⎡ 0 a b p ⎤ ⎫ where F is any
⎪⎪⎨⎢⎢ ⎥ p ∈F ⎪⎪⎬
⎪⎢ 0 u 0 c ⎥ a, b, c, d, u, v,
0 0 v d ⎥ ⎪
⎪⎩⎣⎢ 0 0 0 0 ⎦⎥ ⎭⎪
field. E(R) is multiplicatively closed with four D-classes described as follows:
⎧⎡ 0 m n mp + nq ⎤⎫ ⎧⎡ 0 a 0 ac ⎤⎫ ⎧⎡ 0 0 b bd ⎤⎫
{ }⎪⎨⎪⎪⎪⎩⎢⎢⎢⎢⎣1 0 p ⎥⎥⎥⎦⎥⎪⎭⎪⎪⎪⎬ ⎪⎨⎪⎢⎢ 0 1 0 ⎥⎥⎪⎪⎬ & ⎪⎪⎨⎢⎢ 0 0 ⎥⎥⎬⎪⎪ >
0 0 1 q > ⎪⎢ 0 0 0 c ⎥⎪ ⎪⎢ 0 0 1 0 ⎥⎪ 04×4
0 0 0 0 ⎩⎪⎣⎢ 0 0 0 0 0 0 0 d
0
0 ⎥⎦⎭⎪ ⎪⎩⎢⎣ 0 0 ⎦⎥⎪⎭
where a, b, c, . . . , q vary freely over F. If I and J are the primitive skew lattice ideals determined
by the middle left and middle right D-classes, then QI, QJ and ann(R) = ann(QI) are represented
respectively by
⎡0 a 0 v⎤ ⎡0 0 b v⎤ ⎡0 0 0 v⎤
⎢ ⎥ ⎢ ⎥ ⎢ ⎥
⎢ 0 u 0 c ⎥, ⎢ 0 0 0 0 ⎥, ⎢ 0 0 0 0 ⎥. £
⎢ 0 0 0 0 ⎥ ⎢ 0 0 u d ⎥ ⎢ 0 0 0 0 ⎥
⎣⎢ 0 0 0 0 ⎦⎥ ⎢⎣ 0 0 0 0 ⎥⎦ ⎣⎢ 0 0 0 0 ⎦⎥
Theorem 6.5.3 can be applied repeatedly. Doing so leads to a modification of direct sum
decompositions of rings, which we describe this in full generality, independent of any special
assumption on R and E(R). We begin with a ring R and a set of subrings of R, {Qi⎪i ∈ I}. Let
∑i⊕∈I Qi be the direct sum of the Qi, the subring of the direct product ∏Qi consisting of all I-
tuples with only finitely many non-0 components. Define σ: ∑i⊕∈I Qi → R by σ(⟨xi⎪i ∈ I⟩) = ∑xi.
σ preserves addition. It preserves multiplication precisely when ∑xi∑yi = ∑xiyi holds, which it
does if xiyj = 0 for all i ≠ j. If in addition the image ∑ Qi = R, then each Qi must be an ideal of R.
When R is a sum of ideals Qi where QiQj ={0} for all i ≠ j, we say that R is the orthosum
of the Qi. With σ as above, σ([ ∑i⊕∈I ann(Qi ) ] = ann(R) and σ–1[ann(R)] = ∑i⊕∈I ann(Qi ) . From
this we have:
244