Page 37 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 37
Preliminaries
Finally, a noncommutative lattice is distributive if it satisfies the even weaker pair of
identities:
D4 : a ∧ (b ∨ c) ∧ a = (a ∧ b∧ a) ∨ (a ∧ c ∧ a).
D4ʹ: a ∨ (b ∧ c) ∨ a = (a ∨ b∨ a) ∧ (a ∨ c ∨ a).
Among skew lattices, D4 and D4ʹ play an important role. For instance, skew lattices in
rings are distributive, as are skew Boolean algebras. Moreover, as will be seen in Theorem 3.5.1,
a distributive, noncommutative lattice is a paralattice if and only if it is a quasilattice. It thus
follows that the type of distributivity expressed by D4 and D4ʹ is of maximal general use.
Theorem 1.3.10. Among skew lattices identities D3 and D3 ʹ are independent.
Proof. The skew lattice example of Theorem 1.3.8 satisfies D3 but not D3 ʹ. Switching the ∧ and
∨ tables provides the complementary example satisfying D3 ʹ but not D3. £
Theorem 1.3.11. (Spinks [2000]) Among skew lattices, D4 and D4ʹ are independent.
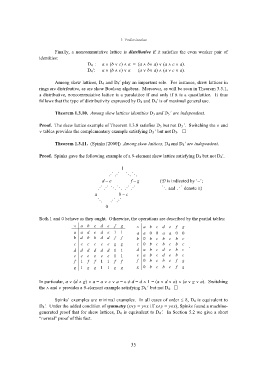
Proof. Spinks gave the following example of a 9-element skew lattice satisfying D4 but not D4ʹ.
1 (D is indicated by ‘–’;
and denote ≥)
d–e f–g
a b–c
0
Both 1 and 0 behave as they ought. Otherwise, the operations are described by the partial tables:
∨abcde f g ∧abcde f g
aad ed e11
bdbbdd f f aa00aa00
b 0bc b c b c
cecceegg
c 0bc bc b c .
dddddd11
eeeeee11 d abcd e b c
f 1 f f 11 f f e abcd e b c
f 0bcbc f g
g1gg11gg
g0bcbc f g
In particular, a ∨ (d ∧ g) ∨ a = a ∨ c ∨ a = e ≠ d = d ∧ 1 = (a ∨ d ∨ a) ∧ (a ∨ g ∨ a). Switching
the ∧ and ∨ provides a 9-element example satisfying D4ʹ but not D4. £
Spinks’ examples are minimal examples. In all cases of order ≤ 8, D4 is equivalent to
D4ʹ. Under the added condition of symmetry (x∨y = y∨x iff x∧y = y∧x), Spinks found a machine-
generated proof that for skew lattices, D4 is equivalent to D4ʹ. In Section 5.2 we give a short
“normal” proof of this fact.
35
Finally, a noncommutative lattice is distributive if it satisfies the even weaker pair of
identities:
D4 : a ∧ (b ∨ c) ∧ a = (a ∧ b∧ a) ∨ (a ∧ c ∧ a).
D4ʹ: a ∨ (b ∧ c) ∨ a = (a ∨ b∨ a) ∧ (a ∨ c ∨ a).
Among skew lattices, D4 and D4ʹ play an important role. For instance, skew lattices in
rings are distributive, as are skew Boolean algebras. Moreover, as will be seen in Theorem 3.5.1,
a distributive, noncommutative lattice is a paralattice if and only if it is a quasilattice. It thus
follows that the type of distributivity expressed by D4 and D4ʹ is of maximal general use.
Theorem 1.3.10. Among skew lattices identities D3 and D3 ʹ are independent.
Proof. The skew lattice example of Theorem 1.3.8 satisfies D3 but not D3 ʹ. Switching the ∧ and
∨ tables provides the complementary example satisfying D3 ʹ but not D3. £
Theorem 1.3.11. (Spinks [2000]) Among skew lattices, D4 and D4ʹ are independent.
Proof. Spinks gave the following example of a 9-element skew lattice satisfying D4 but not D4ʹ.
1 (D is indicated by ‘–’;
and denote ≥)
d–e f–g
a b–c
0
Both 1 and 0 behave as they ought. Otherwise, the operations are described by the partial tables:
∨abcde f g ∧abcde f g
aad ed e11
bdbbdd f f aa00aa00
b 0bc b c b c
cecceegg
c 0bc bc b c .
dddddd11
eeeeee11 d abcd e b c
f 1 f f 11 f f e abcd e b c
f 0bcbc f g
g1gg11gg
g0bcbc f g
In particular, a ∨ (d ∧ g) ∨ a = a ∨ c ∨ a = e ≠ d = d ∧ 1 = (a ∨ d ∨ a) ∧ (a ∨ g ∨ a). Switching
the ∧ and ∨ provides a 9-element example satisfying D4ʹ but not D4. £
Spinks’ examples are minimal examples. In all cases of order ≤ 8, D4 is equivalent to
D4ʹ. Under the added condition of symmetry (x∨y = y∨x iff x∧y = y∧x), Spinks found a machine-
generated proof that for skew lattices, D4 is equivalent to D4ʹ. In Section 5.2 we give a short
“normal” proof of this fact.
35