Page 51 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 51
II: Skew Lattices
Proposition 2.2.3. Any set of mutually commuting elements in a symmetric skew lattice
must generate a sublattice of the skew lattice. £
This is not the case with nonsymmetric skew lattices.
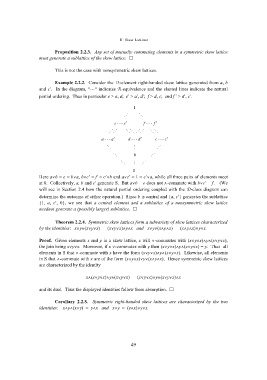
Example 2.2.2. Consider the 13-element right-handed skew lattice generated from a, b
and cʹ. In the diagram, “---“ indicates R-equivalence and the slanted lines indicate the natural
partial ordering. Thus in particular e > a, d; eʹ > aʹ, dʹ; f > d, c; and f ʹ > dʹ, cʹ.
1
e - - - e′ f --- f′
a - - - a′ d --- d′ c - - - c′
b
0
Here a∨b = e = b∨a, b∨cʹ = fʹ = cʹ∨b and a∨cʹ = 1 = cʹ∨a, while all three pairs of elements meet
at 0. Collectively, a, b and cʹ generate S. But a∨b = e does not ∧-commute with b∨cʹ = fʹ. (We
will see in Section 2.4 how the natural partial ordering coupled with the D-class diagram can
determine the outcome of either operation.) Since b is central and {a, cʹ} generates the sublattice
{1, a, cʹ, 0}, we see that a central element and a sublattice of a nonsymmetric skew lattice
neednot generate a (possibly larger) sublattice. £
Theorem 2.2.4. Symmetric skew lattices form a subvariety of skew lattices characterized
by the identities: x∧y∧(x∨y∨x) = (x∨y∨x)∧y∧x and x∨y∨(x∧y∧x) = (x∧y∧x)∨y∨x.
Proof. Given elements x and y in a skew lattice, x will ∨-commutes with (x∨y∨x)∧y∧(x∨y∨x),
the join being x∨y∨x. Moreover, if x ∨-commutes with y then (x∨y∨x)∧y∧(x∨y∨x) = y. Thus all
elements in S that ∨-commute with x have the form (x∨y∨x)∧y∧(x∨y∨x). Likewise, all elements
in S that ∧-commute with x are of the form (x∧y∧x)∨y∨(x∧y∧x). Hence symmetric skew lattices
are characterized by the identity
x∧(x∨y∨x)∧y∧(x∨y∨x) = (x∨y∨x)∧y∧(x∨y∨x)∧x
and its dual. Thus the displayed identities follow from absorption. £
Corollary 2.2.5. Symmetric right-handed skew lattices are characterized by the two
identities: x∧y∧(x∨y) = y∧x and x∨y = (y∧x)∨y∨x.
49
Proposition 2.2.3. Any set of mutually commuting elements in a symmetric skew lattice
must generate a sublattice of the skew lattice. £
This is not the case with nonsymmetric skew lattices.
Example 2.2.2. Consider the 13-element right-handed skew lattice generated from a, b
and cʹ. In the diagram, “---“ indicates R-equivalence and the slanted lines indicate the natural
partial ordering. Thus in particular e > a, d; eʹ > aʹ, dʹ; f > d, c; and f ʹ > dʹ, cʹ.
1
e - - - e′ f --- f′
a - - - a′ d --- d′ c - - - c′
b
0
Here a∨b = e = b∨a, b∨cʹ = fʹ = cʹ∨b and a∨cʹ = 1 = cʹ∨a, while all three pairs of elements meet
at 0. Collectively, a, b and cʹ generate S. But a∨b = e does not ∧-commute with b∨cʹ = fʹ. (We
will see in Section 2.4 how the natural partial ordering coupled with the D-class diagram can
determine the outcome of either operation.) Since b is central and {a, cʹ} generates the sublattice
{1, a, cʹ, 0}, we see that a central element and a sublattice of a nonsymmetric skew lattice
neednot generate a (possibly larger) sublattice. £
Theorem 2.2.4. Symmetric skew lattices form a subvariety of skew lattices characterized
by the identities: x∧y∧(x∨y∨x) = (x∨y∨x)∧y∧x and x∨y∨(x∧y∧x) = (x∧y∧x)∨y∨x.
Proof. Given elements x and y in a skew lattice, x will ∨-commutes with (x∨y∨x)∧y∧(x∨y∨x),
the join being x∨y∨x. Moreover, if x ∨-commutes with y then (x∨y∨x)∧y∧(x∨y∨x) = y. Thus all
elements in S that ∨-commute with x have the form (x∨y∨x)∧y∧(x∨y∨x). Likewise, all elements
in S that ∧-commute with x are of the form (x∧y∧x)∨y∨(x∧y∧x). Hence symmetric skew lattices
are characterized by the identity
x∧(x∨y∨x)∧y∧(x∨y∨x) = (x∨y∨x)∧y∧(x∨y∨x)∧x
and its dual. Thus the displayed identities follow from absorption. £
Corollary 2.2.5. Symmetric right-handed skew lattices are characterized by the two
identities: x∧y∧(x∨y) = y∧x and x∨y = (y∧x)∨y∨x.
49