Page 59 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press

II: Skew Lattices

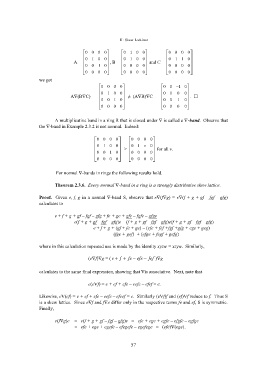
⎡0 0 0 0⎤ ⎡0 1 0 0⎤ ⎡0 0 0 0⎤
⎢0 ⎥ ⎢0 0⎥ ⎢0 0⎥
A = ⎢⎢0 1 0 0 ⎥ , B = ⎢⎢0 1 0 ⎥ and C = ⎢⎢0 1 1 0⎥⎥
0 1 0 ⎥ 0 0 0 ⎥ 0 0

⎢⎣0 0 0 0⎥⎦ ⎣⎢0 0 0 0⎦⎥ ⎢⎣0 0 0 0⎦⎥

we get

⎡0 0 0 0⎤ ⎡0 0 −1 0⎤
⎢0 0⎥ ⎢0 0⎥
A∇(B∇C) = ⎢⎢0 1 0 0⎥⎥ ≠ (A∇B)∇C = ⎢⎢0 1 0 0⎥⎥ . £
0 1 0 1

⎣⎢0 0 0 0⎥⎦ ⎢⎣0 0 0 0⎦⎥

A multiplicative band in a ring R that is closed under ∇ is called a ∇-band. Observe that
the ∇-band in Example 2.3.2 is not normal. Indeed:

⎡0 0 0 0⎤ ⎡0 0 0 0⎤
⎢0 0⎥ ⎢0 0⎥
⎢⎢0 1 0 0⎥⎥ > ⎢⎢0 1 v ⎥ for all v.
0 1 0 0 0 ⎥

⎢⎣0 0 0 0⎦⎥ ⎢⎣0 0 0 0⎦⎥

For normal ∇-bands in rings the following results hold.

Theorem 2.3.6. Every normal ∇-band in a ring is a strongly distributive skew lattice.

Proof. Given e, f, g in a normal ∇-band S, observe that e∇(f∇g) = e∇(f + g + gf – fgf – gfg)
calculates to

e + f + g + gf – fgf – gfg + fe + ge + gfe – fgfe – gfge
– e(f + g + gf – fgf – gfg)e – (f + g + gf – fgf – gfg)e(f + g + gf – fgf – gfg)
= e + f + g + (gf + fe + ge) – (efe + fef +fgf +gfg + ege + geg)
– (fge + gef) + (efge + fegf + gefg)

where in this calculation repeated use is made by the identity xyzw = xzyw. Similarly,

(e∇f)∇g = ( e + f + fe – efe – fef )∇g

calculates to the same final expression, showing that ∇is associative. Next, note that

e(e∇f) = e + ef + efe – eefe – efef = e.

Likewise, e∇(ef) = e + ef + efe – eefe – efeef = e. Similarly (e∇f)f and (ef)∇f reduce to f. Thus S
is a skew lattice. Since e∇f and f∇e differ only in the respective terms fe and ef, S is symmetric.
Finally,

e(f∇g)e = e(f + g + gf – fgf – gfg)e = efe + ege + egfe – efgfe – egfge
= efe + ege + egefe – efegefe – egefege = (efe)∇(ege).

57