Page 82 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 82
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
Elements x and y in a normal skew lattice S are reflections of each other if D-classes
A ≥ B exist such that (i) x and y lie in intermediate D-classes, (ii) ≥ induces an isomorphism of A
with B and (iii) x and y have the same images in A and in B. (This includes the possibility that
either x or y lies in either A or B or both.)
Lemma 2.6.7. Reflection is an equivalence on symmetric, normal skew lattices.
Proof. Let x, y and z be given where a ≥ x ≥ b, a ≥ y ≥ b, c ≥ y ≥ d and c ≥ y ≥ d with a, b, c and d
lying in respective D-classes A, B, C and D such that both A ≅ B and C ≅ D under ≥. It follows
that A ≅ Y and C ≅ Y under ≥ where Y = Dy. Thus A ≅ A∧C ≅ C under ≥ also. Setting J = A∨C,
J is isomorphic to both A and C under ≥ by Theorem 2.6.4. In similar fashion if M = B∧D, then
B and D are isomorphic to M. Hence x, y and z all lie between the D-classes J and M that are
isomorphic under ≥. Clearly x and y and also y and z share a common unique image a∨c up in J
as well as a common unique image b∧d down in M. £
Lemma 2.6.8. Given a symmetric, normal skew lattice, reflection is a congruence.
Proof. If ≥ induces an isomorphism for classes A > B, then for all classes C both A∨C ≅ B∨C
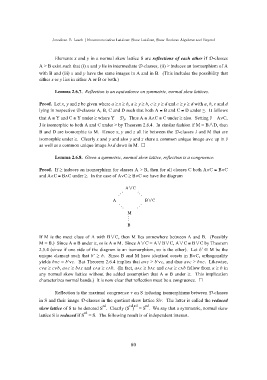
and A∧C ≅ B∧C under ≥. In the case of A∨C ≥ B∨C we have the diagram
A∨C
A B∨C
M
B
If M is the meet class of A with B∨C, then M lies somewhere between A and B. (Possibly
M = B.) Since A ≅ B under ≥, so is A ≅ M. Since A∨C = A∨B∨C, A∨C ≅ B∨C by Theorem
2.5.4 (since if one side of the diagram is an isomorphism, so is the other). Let bʹ ∈ M be the
unique element such that b’ ≥ b. Since B and M have identical cosets in B∨C, orthogonality
yields b∨c = bʹ∨c. But Theorem 2.6.4 implies that a∨c ≥ bʹ∨c, and thus a∨c ≥ b∨c. Likewise,
c∨a ≥ c∨b, a∧c ≥ b∧c and c∧a ≥ c∧b. (In fact, a∧c ≥ b∧c and c∧a ≥ c∧b follow from a ≥ b in
any normal skew lattice without the added assumption that A ≅ B under ≥. This implication
characterizes normal bands.) It is now clear that reflection must be a congruence. £
Reflection is the maximal congruence r on S inducing isomorphisms between D-classes
in S and their image D-classes in the quotient skew lattice S/r. The latter is called the reduced
skew lattice of S to be denoted Srd. Clearly (Srd)rd = Srd. We say that a symmetric, normal skew
lattice S is reduced if Srd = S. The following result is of independent interest.
80
Elements x and y in a normal skew lattice S are reflections of each other if D-classes
A ≥ B exist such that (i) x and y lie in intermediate D-classes, (ii) ≥ induces an isomorphism of A
with B and (iii) x and y have the same images in A and in B. (This includes the possibility that
either x or y lies in either A or B or both.)
Lemma 2.6.7. Reflection is an equivalence on symmetric, normal skew lattices.
Proof. Let x, y and z be given where a ≥ x ≥ b, a ≥ y ≥ b, c ≥ y ≥ d and c ≥ y ≥ d with a, b, c and d
lying in respective D-classes A, B, C and D such that both A ≅ B and C ≅ D under ≥. It follows
that A ≅ Y and C ≅ Y under ≥ where Y = Dy. Thus A ≅ A∧C ≅ C under ≥ also. Setting J = A∨C,
J is isomorphic to both A and C under ≥ by Theorem 2.6.4. In similar fashion if M = B∧D, then
B and D are isomorphic to M. Hence x, y and z all lie between the D-classes J and M that are
isomorphic under ≥. Clearly x and y and also y and z share a common unique image a∨c up in J
as well as a common unique image b∧d down in M. £
Lemma 2.6.8. Given a symmetric, normal skew lattice, reflection is a congruence.
Proof. If ≥ induces an isomorphism for classes A > B, then for all classes C both A∨C ≅ B∨C
and A∧C ≅ B∧C under ≥. In the case of A∨C ≥ B∨C we have the diagram
A∨C
A B∨C
M
B
If M is the meet class of A with B∨C, then M lies somewhere between A and B. (Possibly
M = B.) Since A ≅ B under ≥, so is A ≅ M. Since A∨C = A∨B∨C, A∨C ≅ B∨C by Theorem
2.5.4 (since if one side of the diagram is an isomorphism, so is the other). Let bʹ ∈ M be the
unique element such that b’ ≥ b. Since B and M have identical cosets in B∨C, orthogonality
yields b∨c = bʹ∨c. But Theorem 2.6.4 implies that a∨c ≥ bʹ∨c, and thus a∨c ≥ b∨c. Likewise,
c∨a ≥ c∨b, a∧c ≥ b∧c and c∧a ≥ c∧b. (In fact, a∧c ≥ b∧c and c∧a ≥ c∧b follow from a ≥ b in
any normal skew lattice without the added assumption that A ≅ B under ≥. This implication
characterizes normal bands.) It is now clear that reflection must be a congruence. £
Reflection is the maximal congruence r on S inducing isomorphisms between D-classes
in S and their image D-classes in the quotient skew lattice S/r. The latter is called the reduced
skew lattice of S to be denoted Srd. Clearly (Srd)rd = Srd. We say that a symmetric, normal skew
lattice S is reduced if Srd = S. The following result is of independent interest.
80