Page 132 - Ellingham, Mark, Mariusz Meszka, Primož Moravec, Enes Pasalic, 2014. 2014 PhD Summer School in Discrete Mathematics. Koper: University of Primorska Press. Famnit Lectures, 3.
P. 132
3.5 Nilpotent groups and p -groups
The coclass theorems in particular imply that (p, r ) consists of finitely many maxi-
mal coclass trees and finitely many groups lying outside these trees.
The next results shows that there is a certain kind of periodicity within coclass graphs.
Let S be an infinite pro-p group of coclass r . The subtree (S, k ) of (S) containing all
groups of distance at most k from the main line is called a shaved tree. We denote its
branches by j (S, k ).
Theorem 3.5.41 (Theorem P (du Sautoy, 2001)) Let S be an infinite pro-p group of co-
class r . Then there exist integers d = d ( (S, k )) and f = f ( (S, k )) such that j (S, k ) and
Bj +d (S, k ) are isomorphic as rooted trees for all j ≥ f .
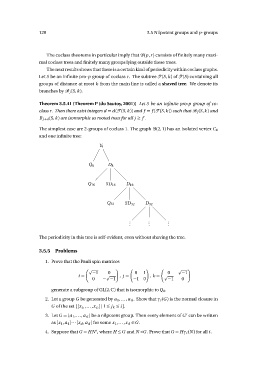
The simplest case are 2-groups of coclass 1. The graph (2, 1) has an isolated vertex C4
and one infinite tree:
V4
Q8 D8
Q16 SD16 D16
Q32 SD32 D32
... ... ...
The periodicity in this tree is self-evident, even without shaving the tree.
3.5.5 Problems
1. Prove that the Pauli spin matrices
i= −1 0 ,j= 0 1 ,k= 0 −1
0 − −1 −1 0 −1 0
generate a subgroup of GL(2, ) that is isomorphic to Q8.
2. Let a group G be generated by a 1, . . . , a d . Show that γi (G ) is the normal closure in
G of the set {[x j1 , . . . , x ji ] | 1 ≤ jk ≤ i }.
3. Let G = 〈a 1, . . . , a d 〉 be a nilpotent group. Then every element of G can be written
as [x1, a 1] · · · [xd , a d ] for some x1, . . . , xd ∈ G .
4. Suppose that G = H N , where H ≤ G and N G . Prove that G = H γi (N ) for all i .
The coclass theorems in particular imply that (p, r ) consists of finitely many maxi-
mal coclass trees and finitely many groups lying outside these trees.
The next results shows that there is a certain kind of periodicity within coclass graphs.
Let S be an infinite pro-p group of coclass r . The subtree (S, k ) of (S) containing all
groups of distance at most k from the main line is called a shaved tree. We denote its
branches by j (S, k ).
Theorem 3.5.41 (Theorem P (du Sautoy, 2001)) Let S be an infinite pro-p group of co-
class r . Then there exist integers d = d ( (S, k )) and f = f ( (S, k )) such that j (S, k ) and
Bj +d (S, k ) are isomorphic as rooted trees for all j ≥ f .
The simplest case are 2-groups of coclass 1. The graph (2, 1) has an isolated vertex C4
and one infinite tree:
V4
Q8 D8
Q16 SD16 D16
Q32 SD32 D32
... ... ...
The periodicity in this tree is self-evident, even without shaving the tree.
3.5.5 Problems
1. Prove that the Pauli spin matrices
i= −1 0 ,j= 0 1 ,k= 0 −1
0 − −1 −1 0 −1 0
generate a subgroup of GL(2, ) that is isomorphic to Q8.
2. Let a group G be generated by a 1, . . . , a d . Show that γi (G ) is the normal closure in
G of the set {[x j1 , . . . , x ji ] | 1 ≤ jk ≤ i }.
3. Let G = 〈a 1, . . . , a d 〉 be a nilpotent group. Then every element of G can be written
as [x1, a 1] · · · [xd , a d ] for some x1, . . . , xd ∈ G .
4. Suppose that G = H N , where H ≤ G and N G . Prove that G = H γi (N ) for all i .