Page 26 - Ellingham, Mark, Mariusz Meszka, Primož Moravec, Enes Pasalic, 2014. 2014 PhD Summer School in Discrete Mathematics. Koper: University of Primorska Press. Famnit Lectures, 3.
P. 26
1.3 Current graphs
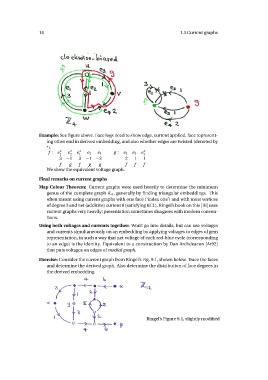
Example: See figure above. Face logs need to show edge, current applied, face represent-
ing other end in derived embedding, and also whether edges are twisted (denoted by
∗). ∗ ∗ ∗ ∗
1 2 1 2
f: e e e e3 e4 g: e4 e3 e
3 −1 3 −1 −2 211
f gf g g fff
We show the equivalent voltage graph.
Final remarks on current graphs
Map Colour Theorem: Current graphs were used heavily to determine the minimum
genus of the complete graph Kn , generally by finding triangular embeddings. This
often meant using current graphs with one face (‘index one’) and with most vertices
of degree 3 and net (additive) current 0 (satisfying KCL). Ringel’s book on this [Ri] uses
current graphs very heavily; presentation sometimes disagrees with modern conven-
tions.
Using both voltages and currents together: Won’t go into details, but can use voltages
and currents simultaneously on an embedding by applying voltages to edges of gem
representation, in such a way that net voltage of each red-blue cycle (corresponding
to an edge) is the identity. Equivalent to a construction by Dan Archdeacon [Ar92]
that puts voltages on edges of medial graph.
Exercise: Consider the current graph from Ringel’s Fig. 9.1, shown below. Trace the faces
and determine the derived graph. Also determine the distribution of face degrees in
the derived embedding.
Ringel’s Figure 9.1, slightly modified
Example: See figure above. Face logs need to show edge, current applied, face represent-
ing other end in derived embedding, and also whether edges are twisted (denoted by
∗). ∗ ∗ ∗ ∗
1 2 1 2
f: e e e e3 e4 g: e4 e3 e
3 −1 3 −1 −2 211
f gf g g fff
We show the equivalent voltage graph.
Final remarks on current graphs
Map Colour Theorem: Current graphs were used heavily to determine the minimum
genus of the complete graph Kn , generally by finding triangular embeddings. This
often meant using current graphs with one face (‘index one’) and with most vertices
of degree 3 and net (additive) current 0 (satisfying KCL). Ringel’s book on this [Ri] uses
current graphs very heavily; presentation sometimes disagrees with modern conven-
tions.
Using both voltages and currents together: Won’t go into details, but can use voltages
and currents simultaneously on an embedding by applying voltages to edges of gem
representation, in such a way that net voltage of each red-blue cycle (corresponding
to an edge) is the identity. Equivalent to a construction by Dan Archdeacon [Ar92]
that puts voltages on edges of medial graph.
Exercise: Consider the current graph from Ringel’s Fig. 9.1, shown below. Trace the faces
and determine the derived graph. Also determine the distribution of face degrees in
the derived embedding.
Ringel’s Figure 9.1, slightly modified