Page 199 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 199
V: Further Topics in Skew Lattices
Thus (ii) and (iii) are equivalent. Their equivalence with (iv) follows from Theorem 5.4.7 above.
Given (ii) – (iv), (iv) forces A > B > C to categorical, since for each component Bʹ in B,
A > Bʹ > C is categorical. Denoting the skew chain by S, (ii) forces S/R and S/L to be
distributive by Lemma 5.6.3 and thus S ⊆ S/R × S/L to be distributive. In light of Theorem 5.4.7,
the final comment is clear. £
Given a > c as above, their midpoint b in component Bʹ depends on the interplay of the
A-cosets and C-cosets within Bʹ. Indeed, given any a ∈ A, the set of images in Bʹ of a is the set
a∧Bʹ∧a = {a∧b∧a⎪b ∈ Bʹ} = {b ∈ Bʹ⎪a > b}. This set parameterizes the A-cosets in Bʹ since
each possesses exactly one b such that a > b. Likewise, for c ∈ C the image set
c∨Bʹ∨c = {c∨b∨c⎪b ∈ Bʹ} = {b ∈ Bʹ⎪b > c}
parameterizes all cosets of C in Bʹ. (See Theorem 2.4.1.) Both images sets are orthogonal in Bʹ
in the following sense. For any a ∈ A, all images of a in Bʹ lie in a unique C-coset in Bʹ.
Likewise for any c ∈ C, all images of c in Bʹ lie in a unique A-coset in Bʹ. Finally, given a > c
with a ∈ A and c ∈ C, their unique midpoint b ∈ Bʹ lies jointly in the C-coset in Bʹ containing all
images of a in Bʹ and in the A-coset in Bʹ containing all images of c in Bʹ. (See Theorem 5.4.6.)
Of course, every b in Bʹ is the midpoint of some pair a > c. For a fixed pair a > c, the set µ(a, c)
of all midpoints in B is a rectangular subalgebra that parameterizes the class of all AC-
components in B: let b in µ(a, c) correspond to the component Bʹ containing b.
∗
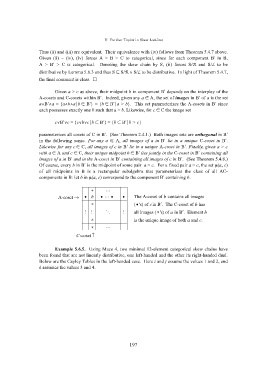
A-coset → • b • • • The A-coset of b contains all images
∗ ( • 's) of c in B′. The C-coset of b has
all images ( ∗ 's) of a in B′. Element b
∗ is the unique image of both a and c.
∗
C-coset ↑
Example 5.6.5. Using Mace 4, two minimal 12-element categorical skew chains have
been found that are not linearly distributive, one left-handed and the other its right-handed dual.
Below are the Cayley Tables in the left-handed case. Here i and j assume the values 1 and 2, and
k assumes the values 3 and 4.
197
Thus (ii) and (iii) are equivalent. Their equivalence with (iv) follows from Theorem 5.4.7 above.
Given (ii) – (iv), (iv) forces A > B > C to categorical, since for each component Bʹ in B,
A > Bʹ > C is categorical. Denoting the skew chain by S, (ii) forces S/R and S/L to be
distributive by Lemma 5.6.3 and thus S ⊆ S/R × S/L to be distributive. In light of Theorem 5.4.7,
the final comment is clear. £
Given a > c as above, their midpoint b in component Bʹ depends on the interplay of the
A-cosets and C-cosets within Bʹ. Indeed, given any a ∈ A, the set of images in Bʹ of a is the set
a∧Bʹ∧a = {a∧b∧a⎪b ∈ Bʹ} = {b ∈ Bʹ⎪a > b}. This set parameterizes the A-cosets in Bʹ since
each possesses exactly one b such that a > b. Likewise, for c ∈ C the image set
c∨Bʹ∨c = {c∨b∨c⎪b ∈ Bʹ} = {b ∈ Bʹ⎪b > c}
parameterizes all cosets of C in Bʹ. (See Theorem 2.4.1.) Both images sets are orthogonal in Bʹ
in the following sense. For any a ∈ A, all images of a in Bʹ lie in a unique C-coset in Bʹ.
Likewise for any c ∈ C, all images of c in Bʹ lie in a unique A-coset in Bʹ. Finally, given a > c
with a ∈ A and c ∈ C, their unique midpoint b ∈ Bʹ lies jointly in the C-coset in Bʹ containing all
images of a in Bʹ and in the A-coset in Bʹ containing all images of c in Bʹ. (See Theorem 5.4.6.)
Of course, every b in Bʹ is the midpoint of some pair a > c. For a fixed pair a > c, the set µ(a, c)
of all midpoints in B is a rectangular subalgebra that parameterizes the class of all AC-
components in B: let b in µ(a, c) correspond to the component Bʹ containing b.
∗
A-coset → • b • • • The A-coset of b contains all images
∗ ( • 's) of c in B′. The C-coset of b has
all images ( ∗ 's) of a in B′. Element b
∗ is the unique image of both a and c.
∗
C-coset ↑
Example 5.6.5. Using Mace 4, two minimal 12-element categorical skew chains have
been found that are not linearly distributive, one left-handed and the other its right-handed dual.
Below are the Cayley Tables in the left-handed case. Here i and j assume the values 1 and 2, and
k assumes the values 3 and 4.
197