Page 204 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 204
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
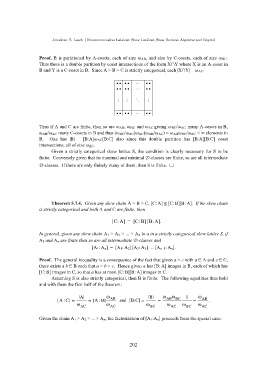
Proof. B is partitioned by A-cosets, each of size ωAB, and also by C-cosets, each of size ωBC.
Thus there is a double partition by coset intersections of the form X∩Y where X is an A-coset in
B and Y is a C-coset in B. Since A > B > C is strictly categorical, each |X∩Y| = ωAC.
•• •• ••
•• •• ••
•• •• ••
Thus if A and C are finite, then so are ωAB, ωBC and ωAC giving ωBC/ωAC many A-cosets in B,
ωAB/ωAC many C-cosets in B and thus (ωBC/ωAC)ωAC(ωAB/ωAC) = ωABωBC/ωAC < ∞ elements in
B. One has |B| = [B:A]ωAC[B:C] also since this double partition has [B:A][B:C] coset
intersections, all of size ωAC.
Given a strictly categorical skew lattice S, the condition is clearly necessary for S to be
finite. Conversely given that its maximal and minimal D-classes are finite, so are all intermediate
D-classes. If there are only finitely many of them, then S is finite. £
Theorem 5.7.4. Given any skew chain A > B > C, [C: A] ≤ [C: B][B: A]. If the skew chain
is strictly categorical and both A and C are finite, then
[C: A] = [C: B] [B: A].
In general, given any skew chain A1 > A2 > ... > An in a in a strictly categorical skew lattice S, if
A1 and An are finite then so are all intermediate D-classes and
[A1: An] = [A1: A2] [A2: A3] … [An–1: An].
Proof. The general inequality is a consequence of the fact that given a > c with a ∈ A and c ∈ C,
there exists a b ∈ B such that a > b > c. Hence given a has [B: A] images in B, each of which has
[C: B] images in C, so that a has at most [C: B][B: A] images in C.
Assuming S is also strictly categorical, then B is finite. The following equalities thus hold
and with them the first half of the theorem:
[A : C] = |A| = [A : B] ωAB and [B:C] = |B| = ωABωBC 1 = ωAB .
ω AC ω AC ω BC ωAC ωBC ωAC
Given the chain A1 > A2 > ... > An, the factorization of [A1:An] proceeds from the special case:
202
Proof. B is partitioned by A-cosets, each of size ωAB, and also by C-cosets, each of size ωBC.
Thus there is a double partition by coset intersections of the form X∩Y where X is an A-coset in
B and Y is a C-coset in B. Since A > B > C is strictly categorical, each |X∩Y| = ωAC.
•• •• ••
•• •• ••
•• •• ••
Thus if A and C are finite, then so are ωAB, ωBC and ωAC giving ωBC/ωAC many A-cosets in B,
ωAB/ωAC many C-cosets in B and thus (ωBC/ωAC)ωAC(ωAB/ωAC) = ωABωBC/ωAC < ∞ elements in
B. One has |B| = [B:A]ωAC[B:C] also since this double partition has [B:A][B:C] coset
intersections, all of size ωAC.
Given a strictly categorical skew lattice S, the condition is clearly necessary for S to be
finite. Conversely given that its maximal and minimal D-classes are finite, so are all intermediate
D-classes. If there are only finitely many of them, then S is finite. £
Theorem 5.7.4. Given any skew chain A > B > C, [C: A] ≤ [C: B][B: A]. If the skew chain
is strictly categorical and both A and C are finite, then
[C: A] = [C: B] [B: A].
In general, given any skew chain A1 > A2 > ... > An in a in a strictly categorical skew lattice S, if
A1 and An are finite then so are all intermediate D-classes and
[A1: An] = [A1: A2] [A2: A3] … [An–1: An].
Proof. The general inequality is a consequence of the fact that given a > c with a ∈ A and c ∈ C,
there exists a b ∈ B such that a > b > c. Hence given a has [B: A] images in B, each of which has
[C: B] images in C, so that a has at most [C: B][B: A] images in C.
Assuming S is also strictly categorical, then B is finite. The following equalities thus hold
and with them the first half of the theorem:
[A : C] = |A| = [A : B] ωAB and [B:C] = |B| = ωABωBC 1 = ωAB .
ω AC ω AC ω BC ωAC ωBC ωAC
Given the chain A1 > A2 > ... > An, the factorization of [A1:An] proceeds from the special case:
202