Page 212 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 212
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
Theorem 6.1.2. Given any naturally totally ordered set of idempotents T in a ring R,
S = e∈T Re is a band that together with ○ forms a right-handed skew lattice in R that is
maximal with respect to being right-handed and containing T as a lattice section. £
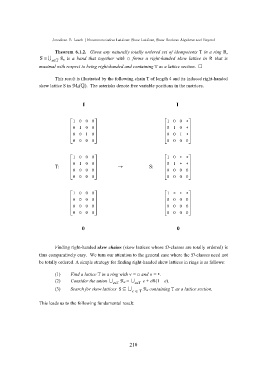
This result is illustrated by the following chain T of length 4 and its induced right-handed
skew lattice S in M4(/). The asterisks denote free variable positions in the matrices.
I I
⎡1 0 0 0⎤ ⎡1 0 0 ∗⎤
⎢0 1 0 0⎥ ⎢0 1 0 ∗⎥
⎢⎢0 ⎥ ⎢⎢0 0 1 ∗⎥⎥
0 1 0 ⎥ ⎣⎢0 0 0 0⎥⎦
⎢⎣0 0 0 0⎥⎦
⎡1 0 0 0⎤ ⎡1 0 ∗ ∗⎤
⎢0 1 0 0⎥ ⎢0 1 ∗ ∗⎥
T: ⎢⎢0 ⎥ → S: ⎢⎢0 0 0 0⎥⎥
0 0 0 ⎥
⎣⎢0 0 0 0⎥⎦ ⎣⎢0 0 0 0⎥⎦
⎡1 0 0 0⎤ ⎡1 ∗ ∗ ∗⎤
⎢0 0 0 0⎥ ⎢0 0 0 0⎥
⎢⎢0 ⎥ ⎢⎢0 0 0 0⎥⎥
0 0 0 ⎥ ⎢⎣0 0 0 0⎦⎥
⎣⎢0 0 0 0⎦⎥
0 0
Finding right-handed skew chains (skew lattices whose D-classes are totally ordered) is
thus comparatively easy. We turn our attention to the general case where the D-classes need not
be totally ordered. A simple strategy for finding right-handed skew lattices in rings is as follows:
(1) Find a lattice T in a ring with ∨ = ○ and ∧ = •.
(2) Consider the union e∈T Re = e∈T e + eR(1 – e).
(3) Search for skew lattices S ⊆ e ∈ T Re containing T as a lattice section.
This leads us to the following fundamental result:
210
Theorem 6.1.2. Given any naturally totally ordered set of idempotents T in a ring R,
S = e∈T Re is a band that together with ○ forms a right-handed skew lattice in R that is
maximal with respect to being right-handed and containing T as a lattice section. £
This result is illustrated by the following chain T of length 4 and its induced right-handed
skew lattice S in M4(/). The asterisks denote free variable positions in the matrices.
I I
⎡1 0 0 0⎤ ⎡1 0 0 ∗⎤
⎢0 1 0 0⎥ ⎢0 1 0 ∗⎥
⎢⎢0 ⎥ ⎢⎢0 0 1 ∗⎥⎥
0 1 0 ⎥ ⎣⎢0 0 0 0⎥⎦
⎢⎣0 0 0 0⎥⎦
⎡1 0 0 0⎤ ⎡1 0 ∗ ∗⎤
⎢0 1 0 0⎥ ⎢0 1 ∗ ∗⎥
T: ⎢⎢0 ⎥ → S: ⎢⎢0 0 0 0⎥⎥
0 0 0 ⎥
⎣⎢0 0 0 0⎥⎦ ⎣⎢0 0 0 0⎥⎦
⎡1 0 0 0⎤ ⎡1 ∗ ∗ ∗⎤
⎢0 0 0 0⎥ ⎢0 0 0 0⎥
⎢⎢0 ⎥ ⎢⎢0 0 0 0⎥⎥
0 0 0 ⎥ ⎢⎣0 0 0 0⎦⎥
⎣⎢0 0 0 0⎦⎥
0 0
Finding right-handed skew chains (skew lattices whose D-classes are totally ordered) is
thus comparatively easy. We turn our attention to the general case where the D-classes need not
be totally ordered. A simple strategy for finding right-handed skew lattices in rings is as follows:
(1) Find a lattice T in a ring with ∨ = ○ and ∧ = •.
(2) Consider the union e∈T Re = e∈T e + eR(1 – e).
(3) Search for skew lattices S ⊆ e ∈ T Re containing T as a lattice section.
This leads us to the following fundamental result:
210