Page 219 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 219
VI: Skew Lattices in Rings
If j ∈supp(1 – e)o then Γ(e, j) is empty by Theorem 6.1.7(ii), while e ∧ ej = 0 by Lemma 5.1.9
and assertion (iv) is seen. Otherwise, if j ∉supp(1 – e)o then by the previous theorem
Γ(e, j) = ∩{supp(e∧f)⎮f ∈ T such that j ∈ supp(f) and e ∧ f ≠ 0}
= supp(e ∧ ∧{ f ∈ T⎮ j ∈ supp(f) and e ∧ f ≠ 0}).
But by Lemma 6.1.9, the latter case is equivalent to asserting that e ∧ ej ≠ 0. Thus in this case,
ej = ∧{ f ∈ T⎮e ∧ f ≠ 0 and j ∈ supp(f)})
so that Γ(e, j) = supp(e ∧ ej ) here also. Thus Γ(e, j) = supp(e ∧ ej ) indeed holds for all
j ∈supp(1 – e) and assertion (iii) is finally seen. £
Given the above definitions in the context of a diagonal lattice T and the matrix ring R,
the class space Γ of T in R is the vector subspace consisting of all matrices A ∈R such that for 1
≤ i ≤ n, in the ith column of A the only nonzero entries occur in the supp( ej ) positions. The class
space of T allows use to express Se for any e ∈ T in the following succinct manner.
Theorem 6.1.11. Given T and R as above with right extension S of T, if Γ is the class
space of T in R, then for each e ∈ T, Se = e + eΓ(1 – e) .
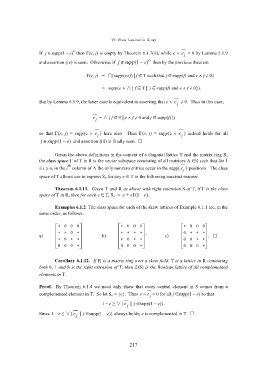
Examples 6.1.2 The class space for each of the skew lattices of Example 6.1.1 are, in the
same order, as follows:
⎡∗ 0 0 0⎤ ⎡∗ 0 0 0⎤ ⎡∗ 0 0 0⎤
⎢∗ ∗ 0 ∗⎥ ⎢∗ ⎥ ⎢0 ∗⎥
a) ⎢⎢∗ 0 ∗ ∗⎥⎥ b) ⎢⎢∗ ∗ ∗ ∗ ⎥ ` c) ⎢⎢0 ∗ ∗ ∗⎥⎥ . £
0 ∗ ∗ ⎥ 0 ∗
⎣⎢0 0 0 ∗⎦⎥ ⎢⎣0 0 0 ∗⎥⎦ ⎢⎣0 0 0 ∗⎦⎥
Corollary 6.1.12. If R is a matrix ring over a skew field, T is a lattice in R containing
both 0, 1 and S is the right extension of T, then Z(S) is the Boolean lattice of all complemented
elements in T.
Proof. By Theorem 6.1.4 we need only show that every central element in S comes from a
complemented element in T. So let Se = {e}. Thus e ∧ ej = 0 for all j ∈supp(1 – e) so that
1 – e ≥ ∨{ ej ⎮ j ∈supp(1 – e)}.
Since 1 – e ≤ ∨{ ej ⎮ j ∈supp(1 – e)} always holds, e is complemented in T. £
217
If j ∈supp(1 – e)o then Γ(e, j) is empty by Theorem 6.1.7(ii), while e ∧ ej = 0 by Lemma 5.1.9
and assertion (iv) is seen. Otherwise, if j ∉supp(1 – e)o then by the previous theorem
Γ(e, j) = ∩{supp(e∧f)⎮f ∈ T such that j ∈ supp(f) and e ∧ f ≠ 0}
= supp(e ∧ ∧{ f ∈ T⎮ j ∈ supp(f) and e ∧ f ≠ 0}).
But by Lemma 6.1.9, the latter case is equivalent to asserting that e ∧ ej ≠ 0. Thus in this case,
ej = ∧{ f ∈ T⎮e ∧ f ≠ 0 and j ∈ supp(f)})
so that Γ(e, j) = supp(e ∧ ej ) here also. Thus Γ(e, j) = supp(e ∧ ej ) indeed holds for all
j ∈supp(1 – e) and assertion (iii) is finally seen. £
Given the above definitions in the context of a diagonal lattice T and the matrix ring R,
the class space Γ of T in R is the vector subspace consisting of all matrices A ∈R such that for 1
≤ i ≤ n, in the ith column of A the only nonzero entries occur in the supp( ej ) positions. The class
space of T allows use to express Se for any e ∈ T in the following succinct manner.
Theorem 6.1.11. Given T and R as above with right extension S of T, if Γ is the class
space of T in R, then for each e ∈ T, Se = e + eΓ(1 – e) .
Examples 6.1.2 The class space for each of the skew lattices of Example 6.1.1 are, in the
same order, as follows:
⎡∗ 0 0 0⎤ ⎡∗ 0 0 0⎤ ⎡∗ 0 0 0⎤
⎢∗ ∗ 0 ∗⎥ ⎢∗ ⎥ ⎢0 ∗⎥
a) ⎢⎢∗ 0 ∗ ∗⎥⎥ b) ⎢⎢∗ ∗ ∗ ∗ ⎥ ` c) ⎢⎢0 ∗ ∗ ∗⎥⎥ . £
0 ∗ ∗ ⎥ 0 ∗
⎣⎢0 0 0 ∗⎦⎥ ⎢⎣0 0 0 ∗⎥⎦ ⎢⎣0 0 0 ∗⎦⎥
Corollary 6.1.12. If R is a matrix ring over a skew field, T is a lattice in R containing
both 0, 1 and S is the right extension of T, then Z(S) is the Boolean lattice of all complemented
elements in T.
Proof. By Theorem 6.1.4 we need only show that every central element in S comes from a
complemented element in T. So let Se = {e}. Thus e ∧ ej = 0 for all j ∈supp(1 – e) so that
1 – e ≥ ∨{ ej ⎮ j ∈supp(1 – e)}.
Since 1 – e ≤ ∨{ ej ⎮ j ∈supp(1 – e)} always holds, e is complemented in T. £
217