Page 228 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 228
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
on T and is somewhat more complex in design than s. Indeed we will soon see that distinct
lattice sections of S can produce different associative joins. But first we have:
Theorem 6.2.12. Given a s-band S with a lattice section T, the binary operation s is
associative if and only if e s f = e ∨T f for all e, f in S, where ∨T is the T-induced associative join
on S. Thus if s is associative, all lattice sections T of S induce a common associative join,
namely s.
Proof. If s equals ∨T for some lattice section T, then clearly s is associative. Conversely, let
s be associative and thus S a skew lattice. The regularity of s plus the fact the xsy = yx in any
D-class of S gives,
esf = (e ste s f s tf) s (te s e s tf s f) = (te s e s tf s f) (e ste s f s tf)
= (etesf tf)(teestf f) = (eL ○ fL)(eR ○ fR) = e ∨T f .
The next-to-last equality is because s reduces to ○ on any left or right regular band in S. The
final assertion of the theorem is clear. £
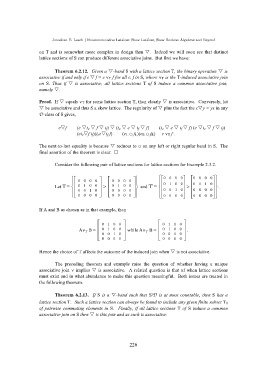
Consider the following pair of lattice sections for lattice sections for Example 2.3.2.
⎧⎡ 0 0 0 0⎤ ⎡0 0 0 0 ⎤⎫ ⎧⎡0 0 0 0⎤ ⎡0 0 0 0⎤⎫
⎨⎪⎩⎪⎢⎢⎢⎣ 0 1 0 1 0 0 ⎦⎥⎥⎥⎭⎪⎬⎪ ⎨⎪⎩⎪⎢⎣⎢⎢000 1 0 0⎥ ⎢0 1 1 000⎦⎥⎥⎥⎬⎪⎭⎪ .
Let T= 0 0 1 0 ⎥ > ⎢ 0 0 0 0 and Tʹ = 0 1 0⎥ > ⎢0 0 0
0 0 0 0 ⎥ ⎢ 0 0 0 0 0 0 0 0
0 ⎥⎦ ⎢⎣ 0 0⎦⎥ ⎢⎣0
If A and B as chosen as in that example, then
⎡0 1 0 0⎤ ⎡0 1 1 0⎤
⎢ ⎥ ⎢ ⎥
A∨T B = ⎢ 0 1 0 0 ⎥ while A∨Tʹ B = ⎢ 0 1 0 0 ⎥ .
0 0 1 0 0 0 1 0
⎣⎢ 0 0 0 0 ⎦⎥ ⎣⎢ 0 0 0 0 ⎥⎦
Hence the choice of T affects the outcome of the induced join when s is not associative.
The preceding theorem and example raise the question of whether having a unique
associative join ∨ implies s is associative. A related question is that of when lattice sections
must exist and in what abundance to make this question meaningful. Both issues are treated in
the following theorem.
Theorem 6.2.13. If S is a s-band such that S/D is at most countable, then S has a
lattice section T. Such a lattice section can always be found to include any given finite subset T0
of pairwise commuting elements in S. Finally, if all lattice sections T of S induce a common
associative join on S then s is this join and as such is associative.
226
on T and is somewhat more complex in design than s. Indeed we will soon see that distinct
lattice sections of S can produce different associative joins. But first we have:
Theorem 6.2.12. Given a s-band S with a lattice section T, the binary operation s is
associative if and only if e s f = e ∨T f for all e, f in S, where ∨T is the T-induced associative join
on S. Thus if s is associative, all lattice sections T of S induce a common associative join,
namely s.
Proof. If s equals ∨T for some lattice section T, then clearly s is associative. Conversely, let
s be associative and thus S a skew lattice. The regularity of s plus the fact the xsy = yx in any
D-class of S gives,
esf = (e ste s f s tf) s (te s e s tf s f) = (te s e s tf s f) (e ste s f s tf)
= (etesf tf)(teestf f) = (eL ○ fL)(eR ○ fR) = e ∨T f .
The next-to-last equality is because s reduces to ○ on any left or right regular band in S. The
final assertion of the theorem is clear. £
Consider the following pair of lattice sections for lattice sections for Example 2.3.2.
⎧⎡ 0 0 0 0⎤ ⎡0 0 0 0 ⎤⎫ ⎧⎡0 0 0 0⎤ ⎡0 0 0 0⎤⎫
⎨⎪⎩⎪⎢⎢⎢⎣ 0 1 0 1 0 0 ⎦⎥⎥⎥⎭⎪⎬⎪ ⎨⎪⎩⎪⎢⎣⎢⎢000 1 0 0⎥ ⎢0 1 1 000⎦⎥⎥⎥⎬⎪⎭⎪ .
Let T= 0 0 1 0 ⎥ > ⎢ 0 0 0 0 and Tʹ = 0 1 0⎥ > ⎢0 0 0
0 0 0 0 ⎥ ⎢ 0 0 0 0 0 0 0 0
0 ⎥⎦ ⎢⎣ 0 0⎦⎥ ⎢⎣0
If A and B as chosen as in that example, then
⎡0 1 0 0⎤ ⎡0 1 1 0⎤
⎢ ⎥ ⎢ ⎥
A∨T B = ⎢ 0 1 0 0 ⎥ while A∨Tʹ B = ⎢ 0 1 0 0 ⎥ .
0 0 1 0 0 0 1 0
⎣⎢ 0 0 0 0 ⎦⎥ ⎣⎢ 0 0 0 0 ⎥⎦
Hence the choice of T affects the outcome of the induced join when s is not associative.
The preceding theorem and example raise the question of whether having a unique
associative join ∨ implies s is associative. A related question is that of when lattice sections
must exist and in what abundance to make this question meaningful. Both issues are treated in
the following theorem.
Theorem 6.2.13. If S is a s-band such that S/D is at most countable, then S has a
lattice section T. Such a lattice section can always be found to include any given finite subset T0
of pairwise commuting elements in S. Finally, if all lattice sections T of S induce a common
associative join on S then s is this join and as such is associative.
226