Page 231 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 231
VI: Skew Lattices in Rings
Proof. That (i) implies (ii) and (iii) follows from Theorem 6.2.8, Lemma 6.2.10 and Theorem
6.2.11. The identities of (ii) and (iii), in their unconditional form, conversely imply (i). Given a,
b, c ∈ P, the only case where the identities need not hold, is the case where a ∈ A and b, c ∈ B.
In all alternative cases these identities hold. We check the case where b ∈ A and c ∈ B. Here we
have b ≻ a, c so that xby = xy whenever x and y are either a or c. Hence
a[b, c]2 = a[bc + cb – bcb – cbc] = ac + acb – acb – ac = 0,
a(bc – cbc) = abc – acbc = ac – ac = 0 = a(bc – cbc)a,
and similarly [b, c]2a = 0 and (bc – bcb)a = 0 = a(bc – bcb)a. The cases b, c ∈ A, or b ∈ B but
c ∈ A are similarly verified, as is the case where a, b, c ∈ B. £
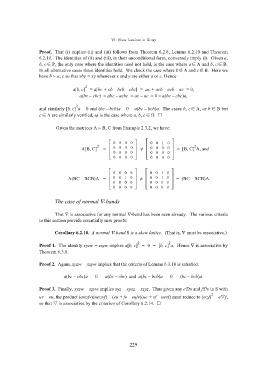
Given the matrices A ≻ B, C from Example 2.3.2, we have:
A[B, C]2 ⎡0 0 0 0⎤ ⎡0 0 1 0⎤ [B, C]2A, and
= ⎢0 0 0 0⎥ ⎢0 0 0 0⎥
0 0 0⎥ ≠ ⎢0 0 0 0⎥ =
⎢0
⎣ 0 0 0 0 ⎦ ⎢⎣ 0 0 0 0 ⎥⎦
⎡0 0 0 0⎤ ⎡0 0 1 0⎤
⎢0 0⎥ ⎢0 0⎥
A(BC – BCB)A = ⎢0 0 1 0⎥ ≠ ⎢0 0 1 0⎥ = (BC – BCB)A.
0 0 0 0
⎢⎣ 0 0 0 0 ⎦⎥ ⎢⎣ 0 0 0 0 ⎥⎦
The case of normal ∇-bands
That ∇ is associative for any normal ∇-band has been seen already. The various criteria
in this section provide essentially new proofs:
Corollary 6.2.18. A normal ∇-band S is a skew lattice. (That is, ∇ must be associative.)
Proof 1. The identity xyzw = xzyw implies a[b, c]2 = 0 = [b, c]2a. Hence ∇ is associative by
Theorem 6.3.8.
Proof 2. Again, xyzw = xzyw implies that the criteria of Lemma 6.3.10 is satisfied:
a(bc – cbc)a = 0 = a(bc – cbc) and a(bc – bcb)a = 0 = (bc – bcb)a.
Proof 3. Finally, xyzw = xzyw implies xyz = xyxz = xzyz. Thus given any eDu and fDv in S with
uv = vu, the product (eu○fv)(ue○vf) = (eu + fv – eufv)(ue + vf – uevf) must reduce to (e○f)2 = esf,
so that s is associative by the criterion of Corollary 6.2.14. £
229
Proof. That (i) implies (ii) and (iii) follows from Theorem 6.2.8, Lemma 6.2.10 and Theorem
6.2.11. The identities of (ii) and (iii), in their unconditional form, conversely imply (i). Given a,
b, c ∈ P, the only case where the identities need not hold, is the case where a ∈ A and b, c ∈ B.
In all alternative cases these identities hold. We check the case where b ∈ A and c ∈ B. Here we
have b ≻ a, c so that xby = xy whenever x and y are either a or c. Hence
a[b, c]2 = a[bc + cb – bcb – cbc] = ac + acb – acb – ac = 0,
a(bc – cbc) = abc – acbc = ac – ac = 0 = a(bc – cbc)a,
and similarly [b, c]2a = 0 and (bc – bcb)a = 0 = a(bc – bcb)a. The cases b, c ∈ A, or b ∈ B but
c ∈ A are similarly verified, as is the case where a, b, c ∈ B. £
Given the matrices A ≻ B, C from Example 2.3.2, we have:
A[B, C]2 ⎡0 0 0 0⎤ ⎡0 0 1 0⎤ [B, C]2A, and
= ⎢0 0 0 0⎥ ⎢0 0 0 0⎥
0 0 0⎥ ≠ ⎢0 0 0 0⎥ =
⎢0
⎣ 0 0 0 0 ⎦ ⎢⎣ 0 0 0 0 ⎥⎦
⎡0 0 0 0⎤ ⎡0 0 1 0⎤
⎢0 0⎥ ⎢0 0⎥
A(BC – BCB)A = ⎢0 0 1 0⎥ ≠ ⎢0 0 1 0⎥ = (BC – BCB)A.
0 0 0 0
⎢⎣ 0 0 0 0 ⎦⎥ ⎢⎣ 0 0 0 0 ⎥⎦
The case of normal ∇-bands
That ∇ is associative for any normal ∇-band has been seen already. The various criteria
in this section provide essentially new proofs:
Corollary 6.2.18. A normal ∇-band S is a skew lattice. (That is, ∇ must be associative.)
Proof 1. The identity xyzw = xzyw implies a[b, c]2 = 0 = [b, c]2a. Hence ∇ is associative by
Theorem 6.3.8.
Proof 2. Again, xyzw = xzyw implies that the criteria of Lemma 6.3.10 is satisfied:
a(bc – cbc)a = 0 = a(bc – cbc) and a(bc – bcb)a = 0 = (bc – bcb)a.
Proof 3. Finally, xyzw = xzyw implies xyz = xyxz = xzyz. Thus given any eDu and fDv in S with
uv = vu, the product (eu○fv)(ue○vf) = (eu + fv – eufv)(ue + vf – uevf) must reduce to (e○f)2 = esf,
so that s is associative by the criterion of Corollary 6.2.14. £
229