Page 65 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 65
II: Skew Lattices
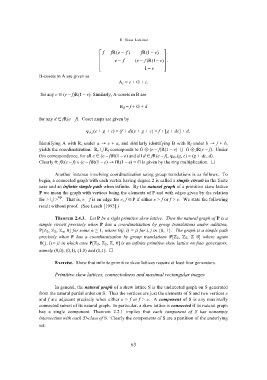
⎡ f fR(e − f ) fR(1 − e) ⎤
⎢ e − f (e − f )R(1 − e)⎥⎥ .
⎢
⎢⎣ 1 − e ⎦⎥
B-cosets in A are given as
Ac = e + G + c.
for any c ∈ (e – f)R(1 – e). Similarly, A-cosets in B are
Bd = f + G + d
for any d ∈ fR(e – f). Coset maps are given by
ϕd,c(e + g + c) = (f + d)(e + g + c) = f + [g + dc] + d.
Identifying A with Re under a → e + a, and similarly identifying B with Rf under b → f + b,
yields the coordinatization. Re ∪ Rf corresponds to G ⊕ (e – f)R(1 – e) ∪ G ⊕ fR(e – f). Under
this correspondence, for all c ∈ (e – f)R(1 – e) and all d ∈ fR(e – f), ϕd,c(g, c) = (g + dc, d).
Clearly θ: fR(e – f) × (e – f)R(1 – e) → fR(1 – e) = G is given by the ring multiplication. £
Another instance involving coordinatization using group translations is as follows. To
begin, a connected graph with each vertex having degree 2 is called a simple circuit in the finite
case and an infinite simple path when infinite. By the natural graph of a primitive skew lattice
P we mean the graph with vertices being the elements of P and with edges given by the relation
for > ∪ >op. That is, e – f is an edge for e, f ∈ P if either e > f or f > e. We state the following
result without proof. (See Leech [1993].)
Theorem 2.4.3. Let P be a right primitive skew lattice. Then the natural graph of P is a
simple circuit precisely when P has a coordinatization by group translations under addition,
P[&2, &2, &n, θ] for some n ≥ 1, where θ(j, i) = ji for i, j in {0, 1}. The graph is a simple path
precisely when P has a coordinatization by group translations P[&2, &2, & θ] where again
θ( j, i) = ji in which case P[&2, &2, &, θ] is an infinite primitive skew lattice on four generators,
namely (0,0), (0,1), (1,0) and (1,1). £
Exercise. Show that infinite primitive skew lattices require at least four generators.
Primitive skew lattices, connectedness and maximal rectangular inages
In general, the natural graph of a skew lattice S is the undirected graph on S generated
from the natural partial order on S. Thus the vertices are just the elements of S and two vertices e
and f are adjacent precisely when either e > f or f > e. A component of S is any maximally
connected subset of its natural graph. In particular, a skew lattice is connected if its natural graph
has a single component. Theorem 2.2.1 implies that each component of S has nonempty
intersection with each D-class of S. Clearly the components of S are a partition of the underlying
set.
63
⎡ f fR(e − f ) fR(1 − e) ⎤
⎢ e − f (e − f )R(1 − e)⎥⎥ .
⎢
⎢⎣ 1 − e ⎦⎥
B-cosets in A are given as
Ac = e + G + c.
for any c ∈ (e – f)R(1 – e). Similarly, A-cosets in B are
Bd = f + G + d
for any d ∈ fR(e – f). Coset maps are given by
ϕd,c(e + g + c) = (f + d)(e + g + c) = f + [g + dc] + d.
Identifying A with Re under a → e + a, and similarly identifying B with Rf under b → f + b,
yields the coordinatization. Re ∪ Rf corresponds to G ⊕ (e – f)R(1 – e) ∪ G ⊕ fR(e – f). Under
this correspondence, for all c ∈ (e – f)R(1 – e) and all d ∈ fR(e – f), ϕd,c(g, c) = (g + dc, d).
Clearly θ: fR(e – f) × (e – f)R(1 – e) → fR(1 – e) = G is given by the ring multiplication. £
Another instance involving coordinatization using group translations is as follows. To
begin, a connected graph with each vertex having degree 2 is called a simple circuit in the finite
case and an infinite simple path when infinite. By the natural graph of a primitive skew lattice
P we mean the graph with vertices being the elements of P and with edges given by the relation
for > ∪ >op. That is, e – f is an edge for e, f ∈ P if either e > f or f > e. We state the following
result without proof. (See Leech [1993].)
Theorem 2.4.3. Let P be a right primitive skew lattice. Then the natural graph of P is a
simple circuit precisely when P has a coordinatization by group translations under addition,
P[&2, &2, &n, θ] for some n ≥ 1, where θ(j, i) = ji for i, j in {0, 1}. The graph is a simple path
precisely when P has a coordinatization by group translations P[&2, &2, & θ] where again
θ( j, i) = ji in which case P[&2, &2, &, θ] is an infinite primitive skew lattice on four generators,
namely (0,0), (0,1), (1,0) and (1,1). £
Exercise. Show that infinite primitive skew lattices require at least four generators.
Primitive skew lattices, connectedness and maximal rectangular inages
In general, the natural graph of a skew lattice S is the undirected graph on S generated
from the natural partial order on S. Thus the vertices are just the elements of S and two vertices e
and f are adjacent precisely when either e > f or f > e. A component of S is any maximally
connected subset of its natural graph. In particular, a skew lattice is connected if its natural graph
has a single component. Theorem 2.2.1 implies that each component of S has nonempty
intersection with each D-class of S. Clearly the components of S are a partition of the underlying
set.
63