Page 68 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 68
athan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
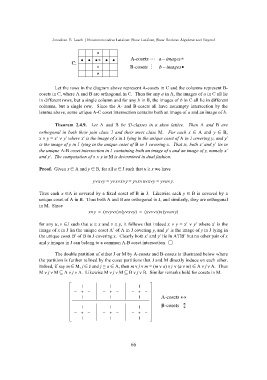
∗ A-cosets a − images∗
C: • • • ∗ • • B-cosets b − images•
∗
∗
Let the rows in the diagram above represent A-cosets in C and the columns represent B-
cosets in C, where A and B are orthogonal in C. Then for any a in A, the images of a in C all lie
in different rows, but a single column and for any b in B, the images of b in C all lie in different
columns, but a single row. Since the A- and B-cosets all have nonempty intersection by the
lemma above, some unique A-C coset intersection contains both an image of a and an image of b.
Theorem 2.4.9. Let A and B be D-classes in a skew lattice. Then A and B are
orthogonal in both their join class J and their meet class M. For each x ∈ A and y ∈ B,
x ∨ y = xʹ ∨ yʹ where xʹ is the image of x in J lying in the unique coset of A in J covering y, and yʹ
is the image of y in J lying in the unique coset of B in J covering x. That is, both xʹ and yʹ lie in
the unique A-B coset intersection in J containing both an image of x and an image of y, namely xʹ
and yʹ. The computation of x ∧ y in M is determined in dual fashion.
Proof. Given x ∈ A and y ∈ B, for all u ∈ J such that u ≥ x we have
y∨x∨y = y∨x∨x∨y = y∨x∨u∨x∨y = y∨u∨y.
Thus each x ∈A is covered by a fixed coset of B in J. Likewise each y ∈ B is covered by a
unique coset of A in B. Thus both A and B are orthogonal in J, and similarly, they are orthogonal
in M. Since
x∨y = (x∨y∨x)∨(y∨x∨y) = (x∨v∨x)∨(y∨u∨y)
for any u, v ∈J such that u ≥ x and v ≥ y, it follows that indeed x ∨ y = xʹ ∨ yʹ where xʹ is the
image of x in J lin the unique coset Aʹ of A in J covering y, and yʹ is the image of y in J lying in
the unique coset Bʹ of B in J covering x. Clearly both xʹ and yʹ lie in Aʹ∩Bʹ but no other pair of x
and y images in J can belong to a common A-B coset intersection. £
The double partition of either J or M by A-cosets and B-cosets is illustrated below where
the partition is further refined by the coset partitions that J and M directly induce on each other.
Indeed, if say m ∈ M, j ∈ J and j ≥ a ∈ A, then m ∨ j ∨ m = (m ∨ a) ∨ j ∨ (a ∨ m) ∈ A ∨ j ∨ A. Thus
M ∨ j ∨ M ⊆ A ∨ j ∨ A. Likewise M ∨ j ∨ M ⊆ B ∨ j ∨ B. Similar remarks hold for cosets in M.
⎡| | |⎤
⎢ −+− ⎥
⎢ − + − − + − ⎥
| | |
⎢ ⎥ A-cosets ↔
⎢ | ⎥ B-cosets
⎢ | −+− | ⎥
⎣⎢ − + − − + − ⎥⎦
| | |
66
∗ A-cosets a − images∗
C: • • • ∗ • • B-cosets b − images•
∗
∗
Let the rows in the diagram above represent A-cosets in C and the columns represent B-
cosets in C, where A and B are orthogonal in C. Then for any a in A, the images of a in C all lie
in different rows, but a single column and for any b in B, the images of b in C all lie in different
columns, but a single row. Since the A- and B-cosets all have nonempty intersection by the
lemma above, some unique A-C coset intersection contains both an image of a and an image of b.
Theorem 2.4.9. Let A and B be D-classes in a skew lattice. Then A and B are
orthogonal in both their join class J and their meet class M. For each x ∈ A and y ∈ B,
x ∨ y = xʹ ∨ yʹ where xʹ is the image of x in J lying in the unique coset of A in J covering y, and yʹ
is the image of y in J lying in the unique coset of B in J covering x. That is, both xʹ and yʹ lie in
the unique A-B coset intersection in J containing both an image of x and an image of y, namely xʹ
and yʹ. The computation of x ∧ y in M is determined in dual fashion.
Proof. Given x ∈ A and y ∈ B, for all u ∈ J such that u ≥ x we have
y∨x∨y = y∨x∨x∨y = y∨x∨u∨x∨y = y∨u∨y.
Thus each x ∈A is covered by a fixed coset of B in J. Likewise each y ∈ B is covered by a
unique coset of A in B. Thus both A and B are orthogonal in J, and similarly, they are orthogonal
in M. Since
x∨y = (x∨y∨x)∨(y∨x∨y) = (x∨v∨x)∨(y∨u∨y)
for any u, v ∈J such that u ≥ x and v ≥ y, it follows that indeed x ∨ y = xʹ ∨ yʹ where xʹ is the
image of x in J lin the unique coset Aʹ of A in J covering y, and yʹ is the image of y in J lying in
the unique coset Bʹ of B in J covering x. Clearly both xʹ and yʹ lie in Aʹ∩Bʹ but no other pair of x
and y images in J can belong to a common A-B coset intersection. £
The double partition of either J or M by A-cosets and B-cosets is illustrated below where
the partition is further refined by the coset partitions that J and M directly induce on each other.
Indeed, if say m ∈ M, j ∈ J and j ≥ a ∈ A, then m ∨ j ∨ m = (m ∨ a) ∨ j ∨ (a ∨ m) ∈ A ∨ j ∨ A. Thus
M ∨ j ∨ M ⊆ A ∨ j ∨ A. Likewise M ∨ j ∨ M ⊆ B ∨ j ∨ B. Similar remarks hold for cosets in M.
⎡| | |⎤
⎢ −+− ⎥
⎢ − + − − + − ⎥
| | |
⎢ ⎥ A-cosets ↔
⎢ | ⎥ B-cosets
⎢ | −+− | ⎥
⎣⎢ − + − − + − ⎥⎦
| | |
66