Page 150 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 150
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
The lattice of subvarieties of skew Boolean ∩-algebras
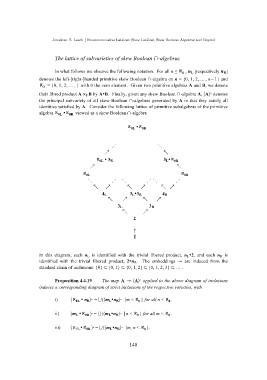
In what follows we observe the following notation. For all n ≤ ℵ0 , nL [respectively nR]
denotes the left-[right-]handed primitive skew Boolean ∩-algebra on n = {0, 1, 2, ... , n – 1} and
ℵ0 = {0, 1, 2, . . . } with 0 the zero element. Given two primitive algebras A and B, we denote
their fibred product A ×2 B by A•B. Finally, given any skew Boolean ∩-algebra A, 〈A〉∩ denotes
the principal subvariety of all skew Boolean ∩-algebras generated by A in that they satisfy all
identities satisfied by A. Consider the following lattice of primitive subalgebras of the primitive
algebra ℵ0L •ℵ0R viewed as a skew Boolean ∩-algebra
ℵ0L •ℵ0R
+,
↗↖
ℵ0L • 3R 3L• ℵ0R
↗ ↖
ℵ0L +, +, ℵ0R
, +
↖↗ ↖↗ ↖↗
4L 3L•3R 4R
↖ ↗↖ ↗
3L 3R
↖ ↗
2
↑
1
In this diagram, each nL is identified with the trivial fibered product, nL•2, and each nR is
identified with the trivial fibered product, 2•nR. The embeddings → are induced from the
standard chain of inclusions: {0} ⊂ {0, 1} ⊂ {0, 1, 2} ⊂ {0, 1, 2, 3} ⊂ … .
Proposition 4.4.19 The map A → 〈A〉∩ applied to the above diagram of inclusions
induces a corresponding diagram of strict inclusions of the respective varieties, with
i) 〈ℵ0L • nR〉∩ = ∪{〈mL•nR〉∩ ⎪m < ℵ0 } for all n < ℵ0 .
ii) 〈mL •ℵ0R 〉∩ = ∪{〈mL•nR〉∩ ⎪n < ℵ0 } for all m < ℵ0 .
iii) 〈ℵ 0L•ℵ0R 〉∩ = ∪{〈mL•nR〉∩ ⎪m, n < ℵ0 }.
148
The lattice of subvarieties of skew Boolean ∩-algebras
In what follows we observe the following notation. For all n ≤ ℵ0 , nL [respectively nR]
denotes the left-[right-]handed primitive skew Boolean ∩-algebra on n = {0, 1, 2, ... , n – 1} and
ℵ0 = {0, 1, 2, . . . } with 0 the zero element. Given two primitive algebras A and B, we denote
their fibred product A ×2 B by A•B. Finally, given any skew Boolean ∩-algebra A, 〈A〉∩ denotes
the principal subvariety of all skew Boolean ∩-algebras generated by A in that they satisfy all
identities satisfied by A. Consider the following lattice of primitive subalgebras of the primitive
algebra ℵ0L •ℵ0R viewed as a skew Boolean ∩-algebra
ℵ0L •ℵ0R
+,
↗↖
ℵ0L • 3R 3L• ℵ0R
↗ ↖
ℵ0L +, +, ℵ0R
, +
↖↗ ↖↗ ↖↗
4L 3L•3R 4R
↖ ↗↖ ↗
3L 3R
↖ ↗
2
↑
1
In this diagram, each nL is identified with the trivial fibered product, nL•2, and each nR is
identified with the trivial fibered product, 2•nR. The embeddings → are induced from the
standard chain of inclusions: {0} ⊂ {0, 1} ⊂ {0, 1, 2} ⊂ {0, 1, 2, 3} ⊂ … .
Proposition 4.4.19 The map A → 〈A〉∩ applied to the above diagram of inclusions
induces a corresponding diagram of strict inclusions of the respective varieties, with
i) 〈ℵ0L • nR〉∩ = ∪{〈mL•nR〉∩ ⎪m < ℵ0 } for all n < ℵ0 .
ii) 〈mL •ℵ0R 〉∩ = ∪{〈mL•nR〉∩ ⎪n < ℵ0 } for all m < ℵ0 .
iii) 〈ℵ 0L•ℵ0R 〉∩ = ∪{〈mL•nR〉∩ ⎪m, n < ℵ0 }.
148