Page 153 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 153
IV: Skew Boolean Algebras
〈3L•3R〉∩
↑
〈3L, 3R〉∩
↖↗ ↖↗
〈3L〉∩ 〈3R〉∩
↖ ↗
〈2〉∩
↑
〈1〉∩
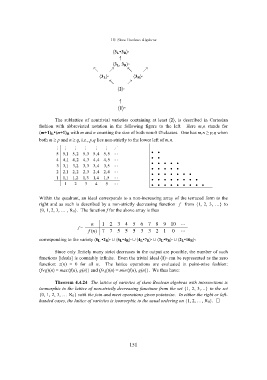
The sublattice of nontrivial varieties containing at least 〈2〉, is described in Cartesian
fashion with abbreviated notation in the following figure to the left. Here m,n stands for
(m+1)L•(n+1)R with m and n counting the size of both non-0 D-classes. One has m,n ≥ p,q when
both m ≥ p and n ≥ q, i.e., p,q lies non-strictly to the lower left of m,n.
••
5 5,1 5, 2 5, 3 5, 4 5, 5 ••
4 4,1 4,2 4, 3 4, 4 4,5 •••••
3 3,1 3, 2 3, 3 3, 4 3, 5 •••••
2 2,1 2, 2 2, 3 2, 4 2, 4 •••••••
1 1,1 1, 2 1, 3 1, 4 1, 5 ••••••••
•••••••••
1 2 3 4 5
Within the quadrant, an ideal corresponds to a non-increasing array of the terraced form to the
right and as such is described by a non-strictly decreasing function f from {1, 2, 3, …} to
{0, 1, 2, 3, … , ℵ0}. The function f for the above array is thus
f= n 1 2 3 4 5 6 7 8 9 10
f (n) 7 7 5 5 5 3 3 2 1 0
corresponding to the variety 〈8L •2R〉∩ ∪ 〈6L•4R〉∩ ∪ 〈4L•7R〉∩ ∪ 〈3L•9R〉∩ ∪ 〈2L•10R〉∩.
Since only finitely many strict decreases in the output are possible, the number of such
functions [ideals] is countably infinite. Even the trivial ideal 〈1〉∩ can be represented as the zero
function: z(n) = 0 for all n. The lattice operations are evaluated in point-wise fashion:
(f∨g)(n) = max{f(n), g(n)} and (f∧g)(n) = min{f(n), g(n)}. We thus have:
Theorem 4.4.24 The lattice of varieties of skew Boolean algebras with intersections is
isomorphic to the lattice of non-strictly decreasing functions from the set {1, 2, 3,…} to the set
{0, 1, 2, 3, … ℵ0} with the join and meet operations given pointwise. In either the right or left-
handed cases, the lattice of varieties is isomorphic to the usual ordering on {1, 2, …, ℵ0}. £
151
〈3L•3R〉∩
↑
〈3L, 3R〉∩
↖↗ ↖↗
〈3L〉∩ 〈3R〉∩
↖ ↗
〈2〉∩
↑
〈1〉∩
The sublattice of nontrivial varieties containing at least 〈2〉, is described in Cartesian
fashion with abbreviated notation in the following figure to the left. Here m,n stands for
(m+1)L•(n+1)R with m and n counting the size of both non-0 D-classes. One has m,n ≥ p,q when
both m ≥ p and n ≥ q, i.e., p,q lies non-strictly to the lower left of m,n.
••
5 5,1 5, 2 5, 3 5, 4 5, 5 ••
4 4,1 4,2 4, 3 4, 4 4,5 •••••
3 3,1 3, 2 3, 3 3, 4 3, 5 •••••
2 2,1 2, 2 2, 3 2, 4 2, 4 •••••••
1 1,1 1, 2 1, 3 1, 4 1, 5 ••••••••
•••••••••
1 2 3 4 5
Within the quadrant, an ideal corresponds to a non-increasing array of the terraced form to the
right and as such is described by a non-strictly decreasing function f from {1, 2, 3, …} to
{0, 1, 2, 3, … , ℵ0}. The function f for the above array is thus
f= n 1 2 3 4 5 6 7 8 9 10
f (n) 7 7 5 5 5 3 3 2 1 0
corresponding to the variety 〈8L •2R〉∩ ∪ 〈6L•4R〉∩ ∪ 〈4L•7R〉∩ ∪ 〈3L•9R〉∩ ∪ 〈2L•10R〉∩.
Since only finitely many strict decreases in the output are possible, the number of such
functions [ideals] is countably infinite. Even the trivial ideal 〈1〉∩ can be represented as the zero
function: z(n) = 0 for all n. The lattice operations are evaluated in point-wise fashion:
(f∨g)(n) = max{f(n), g(n)} and (f∧g)(n) = min{f(n), g(n)}. We thus have:
Theorem 4.4.24 The lattice of varieties of skew Boolean algebras with intersections is
isomorphic to the lattice of non-strictly decreasing functions from the set {1, 2, 3,…} to the set
{0, 1, 2, 3, … ℵ0} with the join and meet operations given pointwise. In either the right or left-
handed cases, the lattice of varieties is isomorphic to the usual ordering on {1, 2, …, ℵ0}. £
151