Page 152 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 152
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
finite power of n+1L•n+1R. Since the given (quasi-)identity holds for all powers of n+1L•n+1R,
it holds for Sʹ and in particular for the assignments, x1 to a1, x2 to a2, … , and xn to an. Since S
and the ai are arbitrary, the theorem follows in the two-sided case. The left-handed and right-
handed cases are similar. £
This above ascending array of principal varieties is only part of the full lattice of all
varieties of skew Boolean ∩-algebras.
Given a partially ordered set P = (P, ≥), an order ideal of P is any nonempty subset I of P
satisfying the following implication: x ∈ I and x ≥ y in P implies y ∈ I also. The following result
of Brian Davey is relevant. (See Theorems 3.3 and 3.5 in [Davey 79].)
Theorem 4.4.22. [Davey 79] Let V be a locally finite, congruence distributive variety.
Then its lattice of subvarieties of V is completely distributive and is isomorphic to the lattice of
order ideals of its partially ordered set of principal subvarieties generated by finite, subdirectly
irreducible algebras and ordered by subvariety inclusion. £
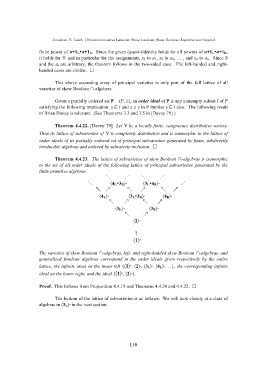
Theorem 4.4.23. The lattice of subvarieties of skew Boolean ∩-algebras is isomorphic
to the set of all order ideals of the following lattice of principal subvarieties generated by the
finite primitive algebras.
, +, +
, 〈4L•3R〉∩ 〈3L•4R〉∩ +
↖↗ ↖↗ ↖↗
〈4L〉∩ 〈3L•3R〉∩ 〈4R〉∩
↖ ↗↖ ↗
〈3L〉∩ 〈3R〉∩
↖ ↗
〈2〉∩
↑
〈1〉∩
The varieties of skew Boolean ∩-algebras, left- and right-handed skew Boolean ∩-algebras, and
generalized Boolean algebras correspond to the order ideals given respectively by the entire
lattice, the infinite ideal on the lower left {〈1〉∩, 〈2〉∩, 〈3L〉∩, 〈4L〉∩, …}, the corresponding infinite
ideal on the lower right, and the ideal {〈1〉∩, 〈2〉∩}.
Proof. This follows from Proposition 4.4.19 and Theorems 4.4.20 and 4.4.22. £
The bottom of the lattice of subvarieties is as follows. We will look closely at a class of
algebras in 〈3L〉∩ in the next section.
150
finite power of n+1L•n+1R. Since the given (quasi-)identity holds for all powers of n+1L•n+1R,
it holds for Sʹ and in particular for the assignments, x1 to a1, x2 to a2, … , and xn to an. Since S
and the ai are arbitrary, the theorem follows in the two-sided case. The left-handed and right-
handed cases are similar. £
This above ascending array of principal varieties is only part of the full lattice of all
varieties of skew Boolean ∩-algebras.
Given a partially ordered set P = (P, ≥), an order ideal of P is any nonempty subset I of P
satisfying the following implication: x ∈ I and x ≥ y in P implies y ∈ I also. The following result
of Brian Davey is relevant. (See Theorems 3.3 and 3.5 in [Davey 79].)
Theorem 4.4.22. [Davey 79] Let V be a locally finite, congruence distributive variety.
Then its lattice of subvarieties of V is completely distributive and is isomorphic to the lattice of
order ideals of its partially ordered set of principal subvarieties generated by finite, subdirectly
irreducible algebras and ordered by subvariety inclusion. £
Theorem 4.4.23. The lattice of subvarieties of skew Boolean ∩-algebras is isomorphic
to the set of all order ideals of the following lattice of principal subvarieties generated by the
finite primitive algebras.
, +, +
, 〈4L•3R〉∩ 〈3L•4R〉∩ +
↖↗ ↖↗ ↖↗
〈4L〉∩ 〈3L•3R〉∩ 〈4R〉∩
↖ ↗↖ ↗
〈3L〉∩ 〈3R〉∩
↖ ↗
〈2〉∩
↑
〈1〉∩
The varieties of skew Boolean ∩-algebras, left- and right-handed skew Boolean ∩-algebras, and
generalized Boolean algebras correspond to the order ideals given respectively by the entire
lattice, the infinite ideal on the lower left {〈1〉∩, 〈2〉∩, 〈3L〉∩, 〈4L〉∩, …}, the corresponding infinite
ideal on the lower right, and the ideal {〈1〉∩, 〈2〉∩}.
Proof. This follows from Proposition 4.4.19 and Theorems 4.4.20 and 4.4.22. £
The bottom of the lattice of subvarieties is as follows. We will look closely at a class of
algebras in 〈3L〉∩ in the next section.
150