Page 174 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 174
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
Dx ≤ Dz. From x ∧ z = y ∧ z we get x ∧ u = y ∧ u for all u in Dz. Thus to prove that x = y it thus
suffices to find u ∈ Dz such that x ≤ u and y ≤ u. Obviously, u = x ∨ z = y ∨ z does the job. On
the other hand, Dz ≤ Dx implies x = x ∨ z ∨ x = x ∨ z = y ∨ z = y ∨ z ∨ y = y. Hence S satisfies
(5.3.1). A similar argument, but with ∨ and ∧ interchanged, shows that (5.3.2) holds. The left-
handed case follows by duality. Since any skew chain S for which S/D is finite is a subalgebra of
S/R × S/L, the general case must follow. £
This leads us to the observation that cancellative skew lattices need not be distributive.
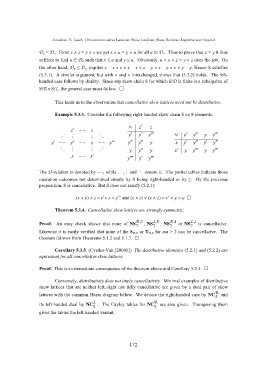
Example 5.3.3. Consider the following right-handed skew chain S on 8 elements.
z′ − − z ∧ z′ z ∨ y′ y′′ y y′′′
y′ y′ y′′′ x y′ y′′ y′ y′′
y′′ y′′ y x′ y y′′′ y y′′′
y′ − − y′′ − − y − − y′′′ y y′′ y
y′′′ y′ y′′′
x − − x′
The D-relation is denoted by – –, while , and denote ≥. The partial tables indicate those
operation outcomes not determined simply by S being right-handed or by ≥. By the previous
proposition, S is cancellative. But S does not satisfy (5.2.1):
(x ∨ y) ∧ z = yʹ∧ z = yʹʹʹ and (x ∧ z) ∨ (y ∧ z) = xʹ ∨ y = y. £
Theorem 5.3.4. Cancellative skew lattices are strongly symmetric.
Proof. An easy check shows that none of NSR7 ,0 , NSL7 ,0 , NSR7 ,1 or NSL7 ,1 is cancellative.
Likewise it is easily verified that none of the Sm,n or Tm,n for mn ≥ 2 can be cancellative. The
theorem follows from Theorems 5.1.2 and 5.1.7. £
Corollary 5.3.5 (Cvetko-Vah [2006b]) The distributive identities (5.2.1) and (5.2.2) are
equivalent for all cancellative skew lattices.
Proof. This is an immediate consequence of the theorem above and Corollary 5.2.4. £
Conversely, distributivity does not imply cancellativity. Minimal examples of distributive
skew lattices that are neither left, right nor fully cancellative are given by a dual pair of skew
lattices with the common Hasse diagram below. We denote the right-handed case by NCR5 and
its left-handed dual by NC5L . The Cayley tables for NCR5 are also given. Transposing them
gives the tables the left-handed variant.
172
Dx ≤ Dz. From x ∧ z = y ∧ z we get x ∧ u = y ∧ u for all u in Dz. Thus to prove that x = y it thus
suffices to find u ∈ Dz such that x ≤ u and y ≤ u. Obviously, u = x ∨ z = y ∨ z does the job. On
the other hand, Dz ≤ Dx implies x = x ∨ z ∨ x = x ∨ z = y ∨ z = y ∨ z ∨ y = y. Hence S satisfies
(5.3.1). A similar argument, but with ∨ and ∧ interchanged, shows that (5.3.2) holds. The left-
handed case follows by duality. Since any skew chain S for which S/D is finite is a subalgebra of
S/R × S/L, the general case must follow. £
This leads us to the observation that cancellative skew lattices need not be distributive.
Example 5.3.3. Consider the following right-handed skew chain S on 8 elements.
z′ − − z ∧ z′ z ∨ y′ y′′ y y′′′
y′ y′ y′′′ x y′ y′′ y′ y′′
y′′ y′′ y x′ y y′′′ y y′′′
y′ − − y′′ − − y − − y′′′ y y′′ y
y′′′ y′ y′′′
x − − x′
The D-relation is denoted by – –, while , and denote ≥. The partial tables indicate those
operation outcomes not determined simply by S being right-handed or by ≥. By the previous
proposition, S is cancellative. But S does not satisfy (5.2.1):
(x ∨ y) ∧ z = yʹ∧ z = yʹʹʹ and (x ∧ z) ∨ (y ∧ z) = xʹ ∨ y = y. £
Theorem 5.3.4. Cancellative skew lattices are strongly symmetric.
Proof. An easy check shows that none of NSR7 ,0 , NSL7 ,0 , NSR7 ,1 or NSL7 ,1 is cancellative.
Likewise it is easily verified that none of the Sm,n or Tm,n for mn ≥ 2 can be cancellative. The
theorem follows from Theorems 5.1.2 and 5.1.7. £
Corollary 5.3.5 (Cvetko-Vah [2006b]) The distributive identities (5.2.1) and (5.2.2) are
equivalent for all cancellative skew lattices.
Proof. This is an immediate consequence of the theorem above and Corollary 5.2.4. £
Conversely, distributivity does not imply cancellativity. Minimal examples of distributive
skew lattices that are neither left, right nor fully cancellative are given by a dual pair of skew
lattices with the common Hasse diagram below. We denote the right-handed case by NCR5 and
its left-handed dual by NC5L . The Cayley tables for NCR5 are also given. Transposing them
gives the tables the left-handed variant.
172