Page 175 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 175
V: Further Topics in Skew Lattices
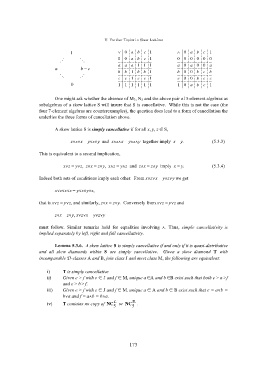
1 ∨ 0 a b c1 ∧0abc1
0 0abc1 000000
a b−c a aa111 a0a00a
b b1bb1 b 00b cb
c c1c c1 c 00b c c
0 1 11111 10abc1
One might ask whether the absence of M3, N5 and the above pair of 5-element algebras as
subalgebras of a skew lattice S will insure that S is cancellative. While this is not the case (the
four 7-element algebras are counterexamples), the question does lead to a form of cancellation the
underlies the three forms of cancellation above.
A skew lattice S is simply cancellative if for all x, y, z ∈ S,
x∨z∨x = y∨z∨y and x∧z∧x = y∧z∧y together imply x = y. (5.3.3)
This is equivalent to a second implication,
x∨z = y∨z, z∨x = z∨y, x∧z = y∧z and z∧x = z∧y imply x = y. (5.3.4)
Indeed both sets of conditions imply each other. From x∨z∨x = y∨z∨y we get
x∨z∨x∨z = y∨z∨y∨z,
that is x∨z = y∨z, and similarly, z∨x = z∨y. Conversely from x∨z = y∨z and
z∨x = z∨y, x∨z∨x = y∨z∨y
must follow. Similar remarks hold for equalities involving ∧. Thus, simple cancellativity is
implied separately by left, right and full cancellativity.
Lemma 5.3.6. A skew lattice S is simply cancellative if and only if it is quasi-distributive
and all skew diamonds within S are simply cancellative. Given a skew diamond T with
incomparable D-classes A and B, join class J and meet class M, the following are equivalent:
i) T is simply cancellative
ii) Given e > f with e ∈ J and f ∈ M, unique a ∈A and b ∈B exist such that both e > a >f
and e > b > f.
iii) Given e > f with e ∈ J and f ∈ M, unique a ∈ A and b ∈ B exist such that e = a∨b =
b∨a and f = a∧b = b∧a.
iv) T contains no copy of NCL5 or NCR5 .
173
1 ∨ 0 a b c1 ∧0abc1
0 0abc1 000000
a b−c a aa111 a0a00a
b b1bb1 b 00b cb
c c1c c1 c 00b c c
0 1 11111 10abc1
One might ask whether the absence of M3, N5 and the above pair of 5-element algebras as
subalgebras of a skew lattice S will insure that S is cancellative. While this is not the case (the
four 7-element algebras are counterexamples), the question does lead to a form of cancellation the
underlies the three forms of cancellation above.
A skew lattice S is simply cancellative if for all x, y, z ∈ S,
x∨z∨x = y∨z∨y and x∧z∧x = y∧z∧y together imply x = y. (5.3.3)
This is equivalent to a second implication,
x∨z = y∨z, z∨x = z∨y, x∧z = y∧z and z∧x = z∧y imply x = y. (5.3.4)
Indeed both sets of conditions imply each other. From x∨z∨x = y∨z∨y we get
x∨z∨x∨z = y∨z∨y∨z,
that is x∨z = y∨z, and similarly, z∨x = z∨y. Conversely from x∨z = y∨z and
z∨x = z∨y, x∨z∨x = y∨z∨y
must follow. Similar remarks hold for equalities involving ∧. Thus, simple cancellativity is
implied separately by left, right and full cancellativity.
Lemma 5.3.6. A skew lattice S is simply cancellative if and only if it is quasi-distributive
and all skew diamonds within S are simply cancellative. Given a skew diamond T with
incomparable D-classes A and B, join class J and meet class M, the following are equivalent:
i) T is simply cancellative
ii) Given e > f with e ∈ J and f ∈ M, unique a ∈A and b ∈B exist such that both e > a >f
and e > b > f.
iii) Given e > f with e ∈ J and f ∈ M, unique a ∈ A and b ∈ B exist such that e = a∨b =
b∨a and f = a∧b = b∧a.
iv) T contains no copy of NCL5 or NCR5 .
173