Page 178 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 178
athan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
Identities (1) and (2) insure that a skew lattice S is simply cancellative (per Theorem 5.3.7) and
(3) ensures that S/L is upper symmetric and S/R is lower symmetric (per Theorem 5.1.2). An
equational base for right cancellative skew lattices is obtained by replacing (3) with the left-right
dual identities. An equational base for cancellative skew lattices is given by replacing (3) with
the two identities for symmetry.
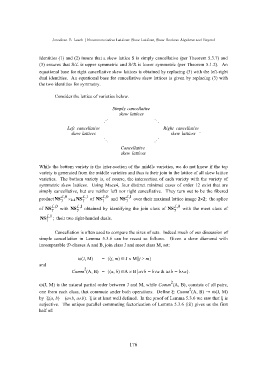
Consider the lattice of varieties below.
Simply cancellative
skew lattices
Left cancellative Right cancellative .
skew lattices skew lattices
Cancellative
skew lattices
While the bottom variety is the inter-section of the middle varieties, we do not know if the top
variety is generated from the middle varieties and thus is their join in the lattice of all skew lattice
varieties. The bottom variety is, of course, the intersection of each variety with the variety of
symmetric skew lattices. Using Mace4, four distinct minimal cases of order 12 exist that are
simply cancellative, but are neither left nor right cancellative. They turn out to be the fibered
product NSL7 ,0 ×2×2 NS L ,1 of NSL7 ,0 and NSL7 ,1 over their maximal lattice image 2×2; the splice
7
of NSL7 ,0 with NSL7 ,1 obtained by identifying the join class of NSL7 ,0 with the meet class of
NSL7 ,1 ; their two right-handed duals.
Cancellation is often used to compare the sizes of sets. Indeed much of our discussion of
simple cancellation in Lemma 5.3.6 can be recast as follows. Given a skew diamond with
incomparable D-classes A and B, join class J and meet class M, set:
ω(J, M) = {(j, m) ∈ J × M⎪j > m}
and
Comm2(A, B) = {(a, b) ∈A × B⎪a∨b = b∨a & a∧b = b∧a}.
ω(J, M) is the natural partial order between J and M, while Comm2(A, B), consists of all pairs,
one from each class, that commute under both operations. Define ξ: Comm2(A, B) → ω(J, M)
by ξ(a, b) = (a∨b, a∧b). ξ is at least well defined. In the proof of Lemma 5.3.6 we saw that ξ is
surjective. The unique parallel commuting factorization of Lemma 5.3.6 (iii) gives us the first
half of:
176
Identities (1) and (2) insure that a skew lattice S is simply cancellative (per Theorem 5.3.7) and
(3) ensures that S/L is upper symmetric and S/R is lower symmetric (per Theorem 5.1.2). An
equational base for right cancellative skew lattices is obtained by replacing (3) with the left-right
dual identities. An equational base for cancellative skew lattices is given by replacing (3) with
the two identities for symmetry.
Consider the lattice of varieties below.
Simply cancellative
skew lattices
Left cancellative Right cancellative .
skew lattices skew lattices
Cancellative
skew lattices
While the bottom variety is the inter-section of the middle varieties, we do not know if the top
variety is generated from the middle varieties and thus is their join in the lattice of all skew lattice
varieties. The bottom variety is, of course, the intersection of each variety with the variety of
symmetric skew lattices. Using Mace4, four distinct minimal cases of order 12 exist that are
simply cancellative, but are neither left nor right cancellative. They turn out to be the fibered
product NSL7 ,0 ×2×2 NS L ,1 of NSL7 ,0 and NSL7 ,1 over their maximal lattice image 2×2; the splice
7
of NSL7 ,0 with NSL7 ,1 obtained by identifying the join class of NSL7 ,0 with the meet class of
NSL7 ,1 ; their two right-handed duals.
Cancellation is often used to compare the sizes of sets. Indeed much of our discussion of
simple cancellation in Lemma 5.3.6 can be recast as follows. Given a skew diamond with
incomparable D-classes A and B, join class J and meet class M, set:
ω(J, M) = {(j, m) ∈ J × M⎪j > m}
and
Comm2(A, B) = {(a, b) ∈A × B⎪a∨b = b∨a & a∧b = b∧a}.
ω(J, M) is the natural partial order between J and M, while Comm2(A, B), consists of all pairs,
one from each class, that commute under both operations. Define ξ: Comm2(A, B) → ω(J, M)
by ξ(a, b) = (a∨b, a∧b). ξ is at least well defined. In the proof of Lemma 5.3.6 we saw that ξ is
surjective. The unique parallel commuting factorization of Lemma 5.3.6 (iii) gives us the first
half of:
176