Page 256 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 256
Jonathan E. Leech │ Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond
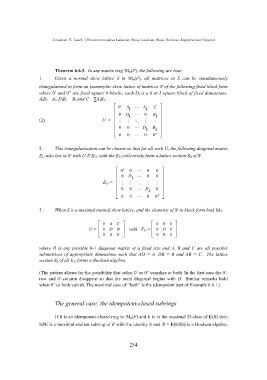
Theorem 6.6.3. In any matrix ring Mn(F), the following are true:
1. Given a normal skew lattice S in Mn(F), all matrices in S can be simultaneously
triangularized to form an isomorphic skew lattice of matrices SΔ of the following fixed block form
where 0ʹ and 0ʺ are fixed square 0-blocks, each Di is a 0 or I square block of fixed dimensions,
AiDi =Ai, DiBi = Bi and C= ∑AiBi:
⎡ 0′ A1 Ak C⎤
⎢ 0 ⎥
(2) U = ⎢ 0 D1 B1 ⎥
⎢
⎢0 ⎥
⎣⎢ 0 ⎥
0 Dk Bk ⎦⎥
0 0 0′′
2. This triangularization can be chosen so that for all such U, the following diagonal matrix
EU also lies in SΔ with U D EU, with the EU collectively form a lattice section S0 of SΔ.
⎡ 0′ 0 0 0 ⎤
⎢0 0⎥
⎢ D1 0 ⎥
EU = ⎢ ⎥
⎢0 0 Dk 0⎥
⎢⎣ 0 0 0 0′′ ⎦⎥
3. When S is a maximal normal skew lattice, and the elements of SΔ in block form look like
⎡0 A C⎤ ⎡0 0 0⎤
U = ⎢ 0 D B ⎥ with EU = ⎢ 0 D 0 ⎥
⎢⎣ 0 0 0 ⎥⎦ ⎣⎢ 0 0 0 ⎥⎦
where D is any possible 0-1 diagonal matrix of a fixed size and A, B and C are all possible
submatrices of appropriate dimensions such that AD = A, DB = B and AB = C. The lattice
section S0 of all EU forms a Boolean algebra.
(The pattern allows for the possibility that either 0ʹ or 0ʺ vanishes or both. In the first case the 0ʹ-
row and 0ʹ-column disappear so that the main diagonal begins with D. Similar remarks hold
when 0ʺ or both vanish. The maximal case of “both” is the idempotent part of Example 6.6.1.)
The general case: the idempotent-closed subrings
If R is an idempotent-closed ring in Mn(F) and E is in the maximal D-class of E(R) then
ERE is a maximal abelian subring of R with the identity E and B = E(ERE) is a Boolean algebra.
254
Theorem 6.6.3. In any matrix ring Mn(F), the following are true:
1. Given a normal skew lattice S in Mn(F), all matrices in S can be simultaneously
triangularized to form an isomorphic skew lattice of matrices SΔ of the following fixed block form
where 0ʹ and 0ʺ are fixed square 0-blocks, each Di is a 0 or I square block of fixed dimensions,
AiDi =Ai, DiBi = Bi and C= ∑AiBi:
⎡ 0′ A1 Ak C⎤
⎢ 0 ⎥
(2) U = ⎢ 0 D1 B1 ⎥
⎢
⎢0 ⎥
⎣⎢ 0 ⎥
0 Dk Bk ⎦⎥
0 0 0′′
2. This triangularization can be chosen so that for all such U, the following diagonal matrix
EU also lies in SΔ with U D EU, with the EU collectively form a lattice section S0 of SΔ.
⎡ 0′ 0 0 0 ⎤
⎢0 0⎥
⎢ D1 0 ⎥
EU = ⎢ ⎥
⎢0 0 Dk 0⎥
⎢⎣ 0 0 0 0′′ ⎦⎥
3. When S is a maximal normal skew lattice, and the elements of SΔ in block form look like
⎡0 A C⎤ ⎡0 0 0⎤
U = ⎢ 0 D B ⎥ with EU = ⎢ 0 D 0 ⎥
⎢⎣ 0 0 0 ⎥⎦ ⎣⎢ 0 0 0 ⎥⎦
where D is any possible 0-1 diagonal matrix of a fixed size and A, B and C are all possible
submatrices of appropriate dimensions such that AD = A, DB = B and AB = C. The lattice
section S0 of all EU forms a Boolean algebra.
(The pattern allows for the possibility that either 0ʹ or 0ʺ vanishes or both. In the first case the 0ʹ-
row and 0ʹ-column disappear so that the main diagonal begins with D. Similar remarks hold
when 0ʺ or both vanish. The maximal case of “both” is the idempotent part of Example 6.6.1.)
The general case: the idempotent-closed subrings
If R is an idempotent-closed ring in Mn(F) and E is in the maximal D-class of E(R) then
ERE is a maximal abelian subring of R with the identity E and B = E(ERE) is a Boolean algebra.
254