Page 259 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 259
VI: Skew Lattices in Rings
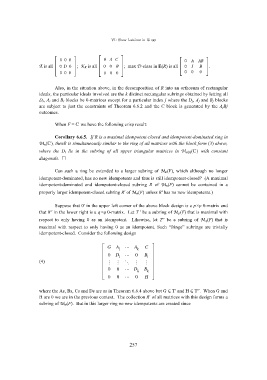
⎡000 ⎤ ⎡0 A C ⎤ ⎡ 0 A AB ⎤
⎢ 0 B ⎥ ⎢ 0 I B ⎥.
A is all ⎢ 0D 0 ⎥ ; KR is all ⎢ 0 0 0 ⎥ ; max D-class in E(R) is all ⎢⎣ 0 0 0 ⎦⎥
⎢ 00 0 ⎥ ⎢⎣ 0
⎣ ⎦ ⎦⎥
Also, in the situation above, in the decomposition of R into an orthosum of rectangular
ideals, the particular ideals involved are the k distinct rectangular subrings obtained by letting all
Di, Ai and Bi blocks be 0-matrices except for a particular index j where the Dj, Aj and Bj blocks
are subject to just the constraints of Theorem 6.6.2 and the C block is generated by the AjBj
outcomes.
When F = 0 we have the following crisp result:
Corollary 6.6.5. If R is a maximal idempotent-closed and idempotent-dominated ring in
Mn(0), thenR is simultaneously similar to the ring of all matrices with the block form (3) above,
where the Di lie in the subring of all upper triangular matrices in Mn(i)(0) with constant
diagonals. £
Can such a ring be extended to a larger subring of Mn(F), which although no longer
idempotent-dominated, has no new idempotents and thus is still idempotent-closed? (A maximal
idempotent-dominated and idempotent-closed subring R of Mn(F) cannot be contained in a
properly larger idempotent-closed subring R′ of Mn(F) unless R′ has no new idempotents.)
Suppose that 0′ in the upper left corner of the above block design is a p×p 0-matrix and
that 0′′ in the lower right is a q×q 0-matrix. Let T ′ be a subring of Mp(F) that is maximal with
respect to only having 0 as an idempotent. Likewise, let T′′ be a subring of Mq(F) that is
maximal with respect to only having 0 as an idempotent. Such “fringe” subrings are trivially
idempotent-closed. Consider the following design
⎡G A1 Ak C⎤
⎢ D1 0 ⎥
⎢0
B1 ⎥
(4) ⎢ ⎥
⎢ ⎥
⎢ 0 0 Dk Bk ⎥
⎣⎢ 0 0 0 H ⎦⎥
where the As, Bs, Cs and Ds are as in Theorem 6.6.4 above but G ∈ T′ and H ∈ T′′. When G and
H are 0 we are in the previous context. The collection Rʹ of all matrices with this design forms a
subring of Mn(F). But in this larger ring no new idempotents are created since
257
⎡000 ⎤ ⎡0 A C ⎤ ⎡ 0 A AB ⎤
⎢ 0 B ⎥ ⎢ 0 I B ⎥.
A is all ⎢ 0D 0 ⎥ ; KR is all ⎢ 0 0 0 ⎥ ; max D-class in E(R) is all ⎢⎣ 0 0 0 ⎦⎥
⎢ 00 0 ⎥ ⎢⎣ 0
⎣ ⎦ ⎦⎥
Also, in the situation above, in the decomposition of R into an orthosum of rectangular
ideals, the particular ideals involved are the k distinct rectangular subrings obtained by letting all
Di, Ai and Bi blocks be 0-matrices except for a particular index j where the Dj, Aj and Bj blocks
are subject to just the constraints of Theorem 6.6.2 and the C block is generated by the AjBj
outcomes.
When F = 0 we have the following crisp result:
Corollary 6.6.5. If R is a maximal idempotent-closed and idempotent-dominated ring in
Mn(0), thenR is simultaneously similar to the ring of all matrices with the block form (3) above,
where the Di lie in the subring of all upper triangular matrices in Mn(i)(0) with constant
diagonals. £
Can such a ring be extended to a larger subring of Mn(F), which although no longer
idempotent-dominated, has no new idempotents and thus is still idempotent-closed? (A maximal
idempotent-dominated and idempotent-closed subring R of Mn(F) cannot be contained in a
properly larger idempotent-closed subring R′ of Mn(F) unless R′ has no new idempotents.)
Suppose that 0′ in the upper left corner of the above block design is a p×p 0-matrix and
that 0′′ in the lower right is a q×q 0-matrix. Let T ′ be a subring of Mp(F) that is maximal with
respect to only having 0 as an idempotent. Likewise, let T′′ be a subring of Mq(F) that is
maximal with respect to only having 0 as an idempotent. Such “fringe” subrings are trivially
idempotent-closed. Consider the following design
⎡G A1 Ak C⎤
⎢ D1 0 ⎥
⎢0
B1 ⎥
(4) ⎢ ⎥
⎢ ⎥
⎢ 0 0 Dk Bk ⎥
⎣⎢ 0 0 0 H ⎦⎥
where the As, Bs, Cs and Ds are as in Theorem 6.6.4 above but G ∈ T′ and H ∈ T′′. When G and
H are 0 we are in the previous context. The collection Rʹ of all matrices with this design forms a
subring of Mn(F). But in this larger ring no new idempotents are created since
257