Page 257 - Leech, Jonathan E. 2020. Noncommutative Lattices: Skew Lattices, Skew Boolean Algebras and Beyond. Koper: University of Primorska Press
P. 257
VI: Skew Lattices in Rings
Let E1, ..., Ek be the atoms of B. Then for all i ≤ k, Ri = EiREi is a ring with identity Ei and no
non-trivial idempotents, and A ≅ R1 ⊕ ··· ⊕ Rk is an idempotent-covered ring with identity E
and E(A) ≅ E1E(R)E1 ⊕ ··· ⊕ EkE(R)Ek as above. We are now ready to state the first of the
two main results in this section.
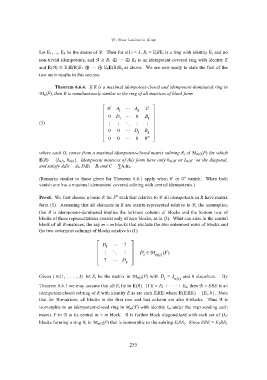
Theorem 6.6.4. If R is a maximal idempotent-closed and idempotent-dominated ring in
Mn(F), then R is simultaneously similar to the ring of all matrices of block form
⎡ 0′ A1 Ak C⎤
⎢ D1 0 ⎥
⎢ 0
B1 ⎥
(3) ⎢ ⎥
⎢ ⎥
⎢ 0 0 Dk Bk ⎥
⎣⎢ 0 0 0 0′′ ⎥⎦
where each Di comes from a maximal idempotent-closed matrix subring Ri of Mn(i)(F) for which
E(R) = {In(i), 0n(i)}. Idempotent matrices of this form have only 0n(i)s or In(i)s on the diagonal,
and satisfy AiDi = Ai, DiBi = Bi and C = ∑AiBi.
(Remarks similar to those given for Theorem 6.6.1 apply when 0ʹ or 0ʺ vanish. When both
vanish one has a maximal idempotent covered subring with central idempotents.)
Proof. We first choose a basis B for Fn such that relative to B all idempotents in R have matrix
form (1). Assuming that all elements in R are matrix-represented relative to B, the assumption
that R is idempotent-dominated implies the leftmost column of blocks and the bottom row of
blocks of these representations consist only of zero blocks, as in (3). What can arise in the central
block of all R-matrices, the say m × m blocks that exclude the two outermost rows of blocks and
the two outermost columns of blocks relative to (1).
⎡ D1 ! ?⎤
⎢ " # ⎥
⎢ " ⎥ Di ∈Mn(i)(F)
⎢ ? ! Dk ⎥
⎣ ⎦
Given i ∈{1, . . . , k} let Ei be the matrix in Mn(i)(F) with Di = In(i) and 0 elsewhere. By
Theorem 6.6.1 we may assume that all Ei lie in E(R). If E = E1 + · · · + Ek, then A = ERE is an
idempotent-closed subring of R with identity E as are each EiREi where E(EiREi) = {Ei, 0}. Note
that for A-matrices, all blocks in the first row and last column are also 0-blocks. Thus A is
isomorphic to an idempotent-closed ring in Mm(F) with identity Im under the map sending each
matrix P in A to its central m × m block. A is further block diagonalized with each set of Di-
blocks forming a ring Ri in Mn(i)(F) that is isomorphic to the subring EiREi. Since ERE = E1RE1
255
Let E1, ..., Ek be the atoms of B. Then for all i ≤ k, Ri = EiREi is a ring with identity Ei and no
non-trivial idempotents, and A ≅ R1 ⊕ ··· ⊕ Rk is an idempotent-covered ring with identity E
and E(A) ≅ E1E(R)E1 ⊕ ··· ⊕ EkE(R)Ek as above. We are now ready to state the first of the
two main results in this section.
Theorem 6.6.4. If R is a maximal idempotent-closed and idempotent-dominated ring in
Mn(F), then R is simultaneously similar to the ring of all matrices of block form
⎡ 0′ A1 Ak C⎤
⎢ D1 0 ⎥
⎢ 0
B1 ⎥
(3) ⎢ ⎥
⎢ ⎥
⎢ 0 0 Dk Bk ⎥
⎣⎢ 0 0 0 0′′ ⎥⎦
where each Di comes from a maximal idempotent-closed matrix subring Ri of Mn(i)(F) for which
E(R) = {In(i), 0n(i)}. Idempotent matrices of this form have only 0n(i)s or In(i)s on the diagonal,
and satisfy AiDi = Ai, DiBi = Bi and C = ∑AiBi.
(Remarks similar to those given for Theorem 6.6.1 apply when 0ʹ or 0ʺ vanish. When both
vanish one has a maximal idempotent covered subring with central idempotents.)
Proof. We first choose a basis B for Fn such that relative to B all idempotents in R have matrix
form (1). Assuming that all elements in R are matrix-represented relative to B, the assumption
that R is idempotent-dominated implies the leftmost column of blocks and the bottom row of
blocks of these representations consist only of zero blocks, as in (3). What can arise in the central
block of all R-matrices, the say m × m blocks that exclude the two outermost rows of blocks and
the two outermost columns of blocks relative to (1).
⎡ D1 ! ?⎤
⎢ " # ⎥
⎢ " ⎥ Di ∈Mn(i)(F)
⎢ ? ! Dk ⎥
⎣ ⎦
Given i ∈{1, . . . , k} let Ei be the matrix in Mn(i)(F) with Di = In(i) and 0 elsewhere. By
Theorem 6.6.1 we may assume that all Ei lie in E(R). If E = E1 + · · · + Ek, then A = ERE is an
idempotent-closed subring of R with identity E as are each EiREi where E(EiREi) = {Ei, 0}. Note
that for A-matrices, all blocks in the first row and last column are also 0-blocks. Thus A is
isomorphic to an idempotent-closed ring in Mm(F) with identity Im under the map sending each
matrix P in A to its central m × m block. A is further block diagonalized with each set of Di-
blocks forming a ring Ri in Mn(i)(F) that is isomorphic to the subring EiREi. Since ERE = E1RE1
255