Page 42 - Fister jr., Iztok, and Andrej Brodnik (eds.). StuCoSReC. Proceedings of the 2018 5th Student Computer Science Research Conference. Koper: University of Primorska Press, 2018
P. 42
ormation, the average FPS was calculated. The results [4] P. Cignoni, F. Ganovelli, E. Gobbetti, F. Marton,
are shown in Table 1. F. Ponchio, R. Scopigno. Planet-sized batched
dynamic adaptive meshes (p-bdam). In Proceedings of
Table 1: Average FPS when rendering a pre-set IEEE Visualization, pages 149–155. IEEE, October
number of triangles 2003.
Number of triangles Average FPS [5] E. Gobbetti, F. Marton, P. Cignoni, M. Di Benedetto,
2 452.90 F. Ganovelli. C-bdam - compressed batched dynamic
8 350.62 adaptive meshes for terrain rendering. Computer
18 397.89 Graphics Forum, 25(3):333–342, December 2006.
32 407.09
50 405.80 [6] J. Schneider and R. Westermann. Gpu-friendly
72 364.40 high-quality terrain rendering. Journal of WSCG,
98 356.11 14(1-3):49–56, February 2006.
128 370.66 [7] T. Schafhitzel, M. Falk, T. Ertl. Real-time rendering
162 382.86 of planets with atmospheres. Journal of WSCG,
200 359.95 15(1-3):91–98, January 2007.
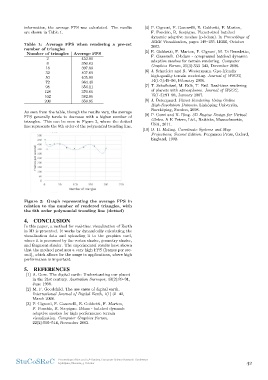
As seen from the table, though the results vary, the average [8] J. O¨ stergaard. Planet Rendering Using Online
FPS generally tends to decrease with a higher number of High-Resolution Datasets. Link¨oping University,
triangles. This can be seen in Figure 2, where the dotted Norrko¨ping, Sweden, 2008.
line represents the 6th order of the polynomial trending line.
[9] P. Cozzi and K. Ring. 3D Engine Design for Virtual
Globes. A K Peters, Ltd., Natticks, Massachusetts,
USA, 2011.
[10] D. H. Maling. Coordinate Systems and Map
Projections, Second Edition. Pergamon Press, Oxford,
England, 1992.
Figure 2: Graph representing the average FPS in 42
relation to the number of rendered triangles, with
the 6th order polynomial trending line (dotted)
4. CONCLUSION
In this paper, a method for real-time visualization of Earth
in 3D is presented. It works by dynamically calculating the
visualization data and uploading it to the graphics card,
where it is processed by the vertex shader, geometry shader,
and fragment shader. The experimental results have shown
that the method produces a very high FPS (frames per sec-
ond), which allows for the usage in applications, where high
performance is important.
5. REFERENCES
[1] A. Gore. The digital earth: Understanding our planet
in the 21st century. Australian Surveyor, 43(2):89–91,
June 1998.
[2] M. F. Goodchild. The use cases of digital earth.
International Journal of Digital Earth, 1(1):31–42,
March 2008.
[3] P. Cignoni, F. Ganovelli, E. Gobbetti, F. Marton,
F. Ponchio, R. Scopigno. Bdam - batched dynamic
adaptive meshes for high performance terrain
visualization. Computer Graphics Forum,
22(2):505–514, November 2003.
StuCoSReC Proceedings of the 2018 5th Student Computer Science Research Conference
Ljubljana, Slovenia, 9 October
are shown in Table 1. F. Ponchio, R. Scopigno. Planet-sized batched
dynamic adaptive meshes (p-bdam). In Proceedings of
Table 1: Average FPS when rendering a pre-set IEEE Visualization, pages 149–155. IEEE, October
number of triangles 2003.
Number of triangles Average FPS [5] E. Gobbetti, F. Marton, P. Cignoni, M. Di Benedetto,
2 452.90 F. Ganovelli. C-bdam - compressed batched dynamic
8 350.62 adaptive meshes for terrain rendering. Computer
18 397.89 Graphics Forum, 25(3):333–342, December 2006.
32 407.09
50 405.80 [6] J. Schneider and R. Westermann. Gpu-friendly
72 364.40 high-quality terrain rendering. Journal of WSCG,
98 356.11 14(1-3):49–56, February 2006.
128 370.66 [7] T. Schafhitzel, M. Falk, T. Ertl. Real-time rendering
162 382.86 of planets with atmospheres. Journal of WSCG,
200 359.95 15(1-3):91–98, January 2007.
As seen from the table, though the results vary, the average [8] J. O¨ stergaard. Planet Rendering Using Online
FPS generally tends to decrease with a higher number of High-Resolution Datasets. Link¨oping University,
triangles. This can be seen in Figure 2, where the dotted Norrko¨ping, Sweden, 2008.
line represents the 6th order of the polynomial trending line.
[9] P. Cozzi and K. Ring. 3D Engine Design for Virtual
Globes. A K Peters, Ltd., Natticks, Massachusetts,
USA, 2011.
[10] D. H. Maling. Coordinate Systems and Map
Projections, Second Edition. Pergamon Press, Oxford,
England, 1992.
Figure 2: Graph representing the average FPS in 42
relation to the number of rendered triangles, with
the 6th order polynomial trending line (dotted)
4. CONCLUSION
In this paper, a method for real-time visualization of Earth
in 3D is presented. It works by dynamically calculating the
visualization data and uploading it to the graphics card,
where it is processed by the vertex shader, geometry shader,
and fragment shader. The experimental results have shown
that the method produces a very high FPS (frames per sec-
ond), which allows for the usage in applications, where high
performance is important.
5. REFERENCES
[1] A. Gore. The digital earth: Understanding our planet
in the 21st century. Australian Surveyor, 43(2):89–91,
June 1998.
[2] M. F. Goodchild. The use cases of digital earth.
International Journal of Digital Earth, 1(1):31–42,
March 2008.
[3] P. Cignoni, F. Ganovelli, E. Gobbetti, F. Marton,
F. Ponchio, R. Scopigno. Bdam - batched dynamic
adaptive meshes for high performance terrain
visualization. Computer Graphics Forum,
22(2):505–514, November 2003.
StuCoSReC Proceedings of the 2018 5th Student Computer Science Research Conference
Ljubljana, Slovenia, 9 October